How many grams of ice at -13°C must be added to 711 grams of water that is initially at a temperature of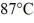 
to produce water at a final temperature of 
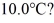Assume that no heat is lost to the surroundings and that the container has negligible mass. The specific heat of liquid water is 4190 J/kg ∙ °C and of ice is 2050 J/kg · °C. For water the normal melting point is 0.00°C and the heat of fusion is 334 × 103 J/kg. The normal boiling point is 100°C and the heat of vaporization is 2.26 × 106 J/kg.
Definitions:
Population Variance
The measure of the dispersion of all values in a population from the population mean.
Population Variance
A measure of the spread or dispersion within a set of data points in a population, indicating how much the data varies from the average.
Dog Food
A type of food specifically formulated and intended for consumption by dogs, made from various ingredients.
Forearm
The part of the arm between the wrist and the elbow.
Q9: A charge Q = -610 nC is
Q11: The graph in the figure shows the
Q14: Four equal negative point charges are located
Q18: When two or more capacitors are connected
Q21: The process shown in the pV diagram
Q25: You want to design an ideal Carnot
Q27: At a certain depth in the ocean,
Q35: A Carnot engine operates between reservoirs at
Q49: The emf and the internal resistance of
Q69: The rotating systems shown in the figure