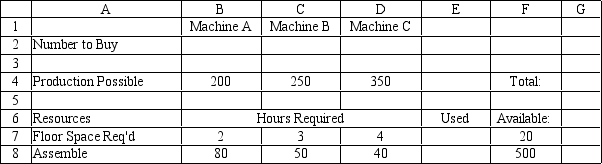
A Company Needs to Purchase Several New Machines to Meet $\quad \mathbf { x } _ { \mathrm { i } } =$

question 3

Essay

A company needs to purchase several new machines to meet its future production needs. It can purchase three different types of machines A, B, and C. Each machine A costs $80,000 and requires 2,000 square feet of floor space. Each machine B costs $50,000 and requires 3,000 square feet of floor space. Each machine C costs $40,000 and requires 5,000 square feet of floor space. The machines can produce 200, 250 and 350 units per day respectively. The plant can only afford $500,000 for all the machines and has at most 20,000 square feet of room for the machines. The company wants to buy as many machines as possible to maximize daily production.
What are the key formulas for this Excel spreadsheet implementation of the following formulation?
Let $\quad \mathbf { x } _ { \mathrm { i } } =$ mumber of machines of type i purchased

$\begin{array}{ll}\text { MAX: } & 200 \mathrm{X}_{1}+250 \mathrm{X}_{2}+300 \mathrm{X}_{3} \\\text { Subject to: } & 2 \mathrm{X}_{1}+3 \mathrm{X}_{2}+5 \mathrm{X}_{3} \leq 20 \\& 80 \mathrm{X}_{1}+50 \mathrm{X}_{2}+40 \mathrm{X}_{3} \leq 500 \\& \mathrm{X}_{1}, \mathrm{X}_{2} \mathrm{X}_{3} \geq 0\end{array}$

---

Definitions:

Catharsis Hypothesis

The idea that expressing or releasing strong emotions leads to relief or emotional cleansing.

Social Exchange Theory

A theory that explains social change and stability as a process of negotiated exchanges between parties, predicting human relationships based on perceived costs and benefits.

Equity Theory

A theory that focuses on the fairness of outcomes that individuals receive in an organization, which influences their motivation and satisfaction.

Mere Exposure Effect

The tendency to prefer stimuli that we've been repeatedly exposed to, regardless of the stimuli's inherent qualities.