TABLE 15-9
Many factors determine the attendance at Major League Baseball games. These factors can include when the game is played, the weather, the opponent, whether or not the team is having a good season, and whether or not a marketing promotion is held. Data from 80 games of the Kansas City Royals for the following variables are collected.
ATTENDANCE = Paid attendance for the game
TEMP = High temperature for the day
WIN% = Team's winning percentage at the time of the game
OPWIN% = Opponent team's winning percentage at the time of the game WEEKEND - 1 if game played on Friday, Saturday or Sunday; 0 otherwise PROMOTION - 1 = if a promotion was held; 0 = if no promotion was held
The regression results using attendance as the dependent variable and the remaining five variables as the independent variables are presented below.
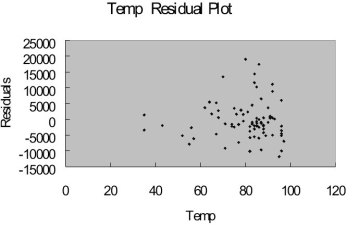
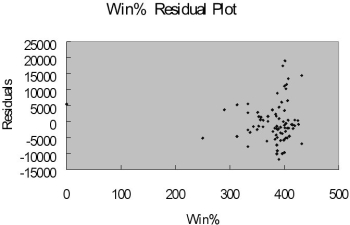
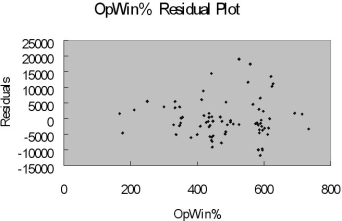
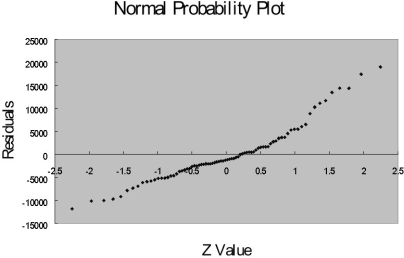
The coefficient of multiple determination ( R 2 j) of each of the 5 predictors with all the other remaining predictors are, respectively, 0.2675, 0.3101, 0.1038, 0.7325, and 0.7308.
-Referring to Table 15-9, what is the value of the test statistic to determine whether PROMOTION makes a significant contribution to the regression model in the presence of the other independent variables at a 5% level of significance?
Definitions:
Decrease
A reduction in size, quantity, or degree of something.
Substitutes
Goods or services that can be used in place of one another, where an increase in the price of one leads to an increase in demand for the other.
Demand Curve
A graphical representation showing the relationship between the price of a good and the quantity demanded by consumers at various prices.
Price
The amount of money required to purchase a good or service, determined by factors such as demand and supply.
Q8: Referring to Table 15-9, which of the
Q28: Referring to Table 15-9, which of the
Q36: Referring to Table 17-5, what is the
Q85: Referring to Table 15-7, suppose the chemist
Q90: Referring to Table 18-5, construct a p
Q101: Referring to Table 14-16, we can conclude
Q115: Referring to Table 16-13, in testing the
Q144: The coefficient of determination represents the ratio
Q196: Referring to Table 13-4, the managers of
Q204: Referring to Table 14-9, what is the