TABLE 13- 11 a Company That Has the Distribution RightsANOVA-Referring to Table 13-11, Which of the Following Assumptions Appears

The Answer of TABLE 13- 11 a Company That Has the Distribution RightsANOVA-Referring to Table 13-11, Which of the Following Assumptions Appears

TABLE 13- 11 a Company That Has the Distribution RightsANOVA-Referring to Table 13-11, Which of the Following Assumptions Appears

The Answer of TABLE 13- 11 a Company That Has the Distribution RightsANOVA-Referring to Table 13-11, Which of the Following Assumptions Appears

Solved

TABLE 13- 11
a Company That Has the Distribution Rights

question 6

Multiple Choice

TABLE 13- 11
A company that has the distribution rights to home video sales of previously released movies would like to use the box office gross (in millions of dollars) to estimate the number of units (in thousands of units) that it can expect to sell. Following is the output from a simple linear regression along with the residual plot and normal probability plot obtained from a data set of 30 different movie titles:
$\begin{array}{l}\text { Regression Statistics }\\\begin{array} { l c } \hline \text { Multiple R } & 0.8531 \\\text { RSquare } & 0.7278 \\\text { Adjusted R Square } & 0.7180 \\\text { Standard Error } & 47.8668 \\\text { Observations } & 30\end{array}\end{array}$
ANOVA
$\begin{array}{lrrrrr}\hline &\text { d f}& \text { SS } & \text { MS } & \text {F }& \text {Significance F} \\\hline \text {Regression }& 1 & 171499.78 & 171499.78 & 74.8505 & 2.1259E-09 \\\text {Residual} & 28 & 64154.42 & 2291.23 & & \\\text {Total} & 29 & 235654.20 & & & \\\hline\end{array}$

$\begin{array}{lrrrrrr}\hline & \text {Coefficients }& \text { Standard Error}& \text { t Stat }& \text { p -value }& \text {Lower 95\% }& \text {Upper 95\% }\\\hline \text { Intercept }& 76.5351 & 11.8318 & 6.4686 & 5.24 \mathrm{E}-07& 52.2987 & 100.7716 \\ \text {Gross} & 4.3331 & 0.5008 & 8.6516 & 2.13 \mathrm{E}-09 & 3.3072 & 5.3590 \\\hline\end{array}$

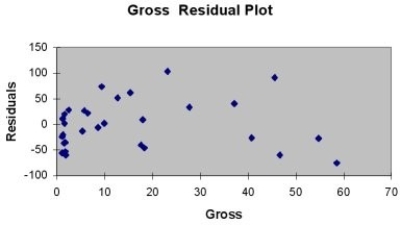
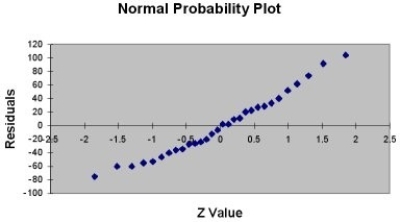
$TABLE 13- 11 A company that has the distribution rights to home video sales of previously released movies would like to use the box office gross (in millions of dollars) to estimate the number of units (in thousands of units) that it can expect to sell. Following is the output from a simple linear regression along with the residual plot and normal probability plot obtained from a data set of 30 different movie titles: \begin{array}{l} \text { Regression Statistics }\\ \begin{array} { l c } \hline \text { Multiple R } & 0.8531 \\ \text { RSquare } & 0.7278 \\ \text { Adjusted R Square } & 0.7180 \\ \text { Standard Error } & 47.8668 \\ \text { Observations } & 30 \end{array} \end{array} ANOVA \begin{array}{lrrrrr} \hline &\text { d f}& \text { SS } & \text { MS } & \text {F }& \text {Significance F} \\ \hline \text {Regression }& 1 & 171499.78 & 171499.78 & 74.8505 & 2.1259E-09 \\ \text {Residual} & 28 & 64154.42 & 2291.23 & & \\ \text {Total} & 29 & 235654.20 & & & \\ \hline\end{array} \begin{array}{lrrrrrr} \hline & \text {Coefficients }& \text { Standard Error}& \text { t Stat }& \text { p -value }& \text {Lower 95\% }& \text {Upper 95\% }\\ \hline \text { Intercept }& 76.5351 & 11.8318 & 6.4686 & 5.24 \mathrm{E}-07& 52.2987 & 100.7716 \\ \text {Gross} & 4.3331 & 0.5008 & 8.6516 & 2.13 \mathrm{E}-09 & 3.3072 & 5.3590 \\ \hline \end{array} -Referring to Table 13-11, which of the following assumptions appears to have been violated? A) homoscedasticity B) independence of errors C) normality of error D) none of the above$ $TABLE 13- 11 A company that has the distribution rights to home video sales of previously released movies would like to use the box office gross (in millions of dollars) to estimate the number of units (in thousands of units) that it can expect to sell. Following is the output from a simple linear regression along with the residual plot and normal probability plot obtained from a data set of 30 different movie titles: \begin{array}{l} \text { Regression Statistics }\\ \begin{array} { l c } \hline \text { Multiple R } & 0.8531 \\ \text { RSquare } & 0.7278 \\ \text { Adjusted R Square } & 0.7180 \\ \text { Standard Error } & 47.8668 \\ \text { Observations } & 30 \end{array} \end{array} ANOVA \begin{array}{lrrrrr} \hline &\text { d f}& \text { SS } & \text { MS } & \text {F }& \text {Significance F} \\ \hline \text {Regression }& 1 & 171499.78 & 171499.78 & 74.8505 & 2.1259E-09 \\ \text {Residual} & 28 & 64154.42 & 2291.23 & & \\ \text {Total} & 29 & 235654.20 & & & \\ \hline\end{array} \begin{array}{lrrrrrr} \hline & \text {Coefficients }& \text { Standard Error}& \text { t Stat }& \text { p -value }& \text {Lower 95\% }& \text {Upper 95\% }\\ \hline \text { Intercept }& 76.5351 & 11.8318 & 6.4686 & 5.24 \mathrm{E}-07& 52.2987 & 100.7716 \\ \text {Gross} & 4.3331 & 0.5008 & 8.6516 & 2.13 \mathrm{E}-09 & 3.3072 & 5.3590 \\ \hline \end{array} -Referring to Table 13-11, which of the following assumptions appears to have been violated? A) homoscedasticity B) independence of errors C) normality of error D) none of the above$
-Referring to Table 13-11, which of the following assumptions appears to have been violated?

Definitions:

Single-Price Monopoly

A market condition where a monopolist sets one price for all consumers of its product, without price discrimination.

Price Elasticity

Measurement of consumer demand variations for a good due to alterations in its price, signifying the degree of consumer sensitivity to these changes.

Discount Coupons

Discount coupons are vouchers that offer a reduction in price for specific items or services, encouraging consumers to make purchases.

Redeem Coupons

The process of exchanging a coupon for a discount, rebate, or any other promotional offer while purchasing a product or service.