TABLE 13- 11
A company that has the distribution rights to home video sales of previously released movies would like to use the box office gross (in millions of dollars) to estimate the number of units (in thousands of units) that it can expect to sell. Following is the output from a simple linear regression along with the residual plot and normal probability plot obtained from a data set of 30 different movie titles:
ANOVA
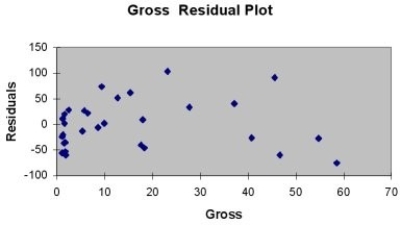
-Referring to Table 13-11, what are, respectively, the lower and upper limits of the 95% confidence interval estimate for the average change in video unit sales as a result of a one million dollars increase in box office?
Definitions:
Condenser
A device used to condense a substance from its gaseous to liquid state by cooling.
Foreign Objects
Items or substances present in parts of the body where they do not naturally belong, potentially causing harm.
Specimen
A sample taken from the body, like blood or tissue, that is tested or studied to understand more about a health condition.
Workforce Drug Testing
The process of screening employees or job applicants for the use of illegal drugs or misuse of prescription medication.
Q3: Referring to Table 12-13, what is the
Q13: Referring to Table 12-10, the expected cell
Q13: Referring to Table 15-8, the quadratic effect
Q24: Referring to Table 14-4, what is the
Q72: Referring to Table 15-9, what is the
Q88: Referring to Table 11-5, what degrees of
Q95: Referring to Table 10-4, what is an
Q123: Referring to Table 13-4, the managers of
Q145: In testing the difference between two proportions
Q255: Referring to Table 14-10, to test the