TABLE 13- 11 a Company That Has the Distribution RightsANOVA-Referring to Table 13-11, the Null Hypothesis for Testing Whether

The Answer of TABLE 13- 11 a Company That Has the Distribution RightsANOVA-Referring to Table 13-11, the Null Hypothesis for Testing Whether

TABLE 13- 11 a Company That Has the Distribution RightsANOVA-Referring to Table 13-11, the Null Hypothesis for Testing Whether

The Answer of TABLE 13- 11 a Company That Has the Distribution RightsANOVA-Referring to Table 13-11, the Null Hypothesis for Testing Whether

Solved

TABLE 13- 11
a Company That Has the Distribution Rights

question 150

True/False

TABLE 13- 11
A company that has the distribution rights to home video sales of previously released movies would like to use the box office gross (in millions of dollars) to estimate the number of units (in thousands of units) that it can expect to sell. Following is the output from a simple linear regression along with the residual plot and normal probability plot obtained from a data set of 30 different movie titles:
$\begin{array}{l}\text { Regression Statistics }\\\begin{array} { l c } \hline \text { Multiple R } & 0.8531 \\\text { RSquare } & 0.7278 \\\text { Adjusted R Square } & 0.7180 \\\text { Standard Error } & 47.8668 \\\text { Observations } & 30\end{array}\end{array}$
ANOVA
$\begin{array}{lrrrrr}\hline &\text { d f}& \text { SS } & \text { MS } & \text {F }& \text {Significance F} \\\hline \text {Regression }& 1 & 171499.78 & 171499.78 & 74.8505 & 2.1259E-09 \\\text {Residual} & 28 & 64154.42 & 2291.23 & & \\\text {Total} & 29 & 235654.20 & & & \\\hline\end{array}$

$\begin{array}{lrrrrrr}\hline & \text {Coefficients }& \text { Standard Error}& \text { t Stat }& \text { p -value }& \text {Lower 95\% }& \text {Upper 95\% }\\\hline \text { Intercept }& 76.5351 & 11.8318 & 6.4686 & 5.24 \mathrm{E}-07& 52.2987 & 100.7716 \\ \text {Gross} & 4.3331 & 0.5008 & 8.6516 & 2.13 \mathrm{E}-09 & 3.3072 & 5.3590 \\\hline\end{array}$

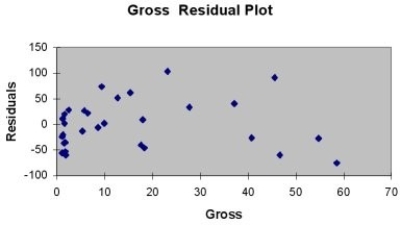
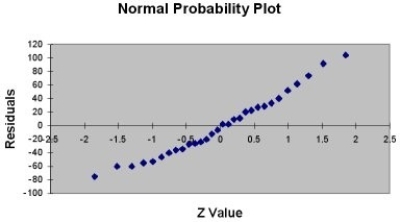
$TABLE 13- 11 A company that has the distribution rights to home video sales of previously released movies would like to use the box office gross (in millions of dollars) to estimate the number of units (in thousands of units) that it can expect to sell. Following is the output from a simple linear regression along with the residual plot and normal probability plot obtained from a data set of 30 different movie titles: \begin{array}{l} \text { Regression Statistics }\\ \begin{array} { l c } \hline \text { Multiple R } & 0.8531 \\ \text { RSquare } & 0.7278 \\ \text { Adjusted R Square } & 0.7180 \\ \text { Standard Error } & 47.8668 \\ \text { Observations } & 30 \end{array} \end{array} ANOVA \begin{array}{lrrrrr} \hline &\text { d f}& \text { SS } & \text { MS } & \text {F }& \text {Significance F} \\ \hline \text {Regression }& 1 & 171499.78 & 171499.78 & 74.8505 & 2.1259E-09 \\ \text {Residual} & 28 & 64154.42 & 2291.23 & & \\ \text {Total} & 29 & 235654.20 & & & \\ \hline\end{array} \begin{array}{lrrrrrr} \hline & \text {Coefficients }& \text { Standard Error}& \text { t Stat }& \text { p -value }& \text {Lower 95\% }& \text {Upper 95\% }\\ \hline \text { Intercept }& 76.5351 & 11.8318 & 6.4686 & 5.24 \mathrm{E}-07& 52.2987 & 100.7716 \\ \text {Gross} & 4.3331 & 0.5008 & 8.6516 & 2.13 \mathrm{E}-09 & 3.3072 & 5.3590 \\ \hline \end{array} -Referring to Table 13-11, the null hypothesis for testing whether there is a linear relationship between box office gross and home video unit sales is There is no linear relationship between box office gross and home video unit sales.$ $TABLE 13- 11 A company that has the distribution rights to home video sales of previously released movies would like to use the box office gross (in millions of dollars) to estimate the number of units (in thousands of units) that it can expect to sell. Following is the output from a simple linear regression along with the residual plot and normal probability plot obtained from a data set of 30 different movie titles: \begin{array}{l} \text { Regression Statistics }\\ \begin{array} { l c } \hline \text { Multiple R } & 0.8531 \\ \text { RSquare } & 0.7278 \\ \text { Adjusted R Square } & 0.7180 \\ \text { Standard Error } & 47.8668 \\ \text { Observations } & 30 \end{array} \end{array} ANOVA \begin{array}{lrrrrr} \hline &\text { d f}& \text { SS } & \text { MS } & \text {F }& \text {Significance F} \\ \hline \text {Regression }& 1 & 171499.78 & 171499.78 & 74.8505 & 2.1259E-09 \\ \text {Residual} & 28 & 64154.42 & 2291.23 & & \\ \text {Total} & 29 & 235654.20 & & & \\ \hline\end{array} \begin{array}{lrrrrrr} \hline & \text {Coefficients }& \text { Standard Error}& \text { t Stat }& \text { p -value }& \text {Lower 95\% }& \text {Upper 95\% }\\ \hline \text { Intercept }& 76.5351 & 11.8318 & 6.4686 & 5.24 \mathrm{E}-07& 52.2987 & 100.7716 \\ \text {Gross} & 4.3331 & 0.5008 & 8.6516 & 2.13 \mathrm{E}-09 & 3.3072 & 5.3590 \\ \hline \end{array} -Referring to Table 13-11, the null hypothesis for testing whether there is a linear relationship between box office gross and home video unit sales is There is no linear relationship between box office gross and home video unit sales.$

-Referring to Table 13-11, the null hypothesis for testing whether there is a linear relationship between box office gross and home video unit sales is "There is no linear relationship between box office gross and home video unit sales."

Explain how companies manage reported earnings per share and the factors influencing such management.

Analyze the effects of corporate actions (stock dividends, stock splits) on shareholders' equity.

Recognize the reasons behind stock repurchases and their financial statement implications.

Understand the allocation of share-based compensation costs and its reporting requirements.

Definitions:

Light Beam

A directed, narrow stream of light photons, which can be visible or invisible and used in various applications, from illumination to communication.

Strength

The quality or state of being physically strong, or the capacity to exert force on physical objects.

Health Of The Ear

The overall condition and functioning of the ear, including the ability to hear and maintain balance, as well as the absence of infection or disease.

Medical Doctor

A qualified individual who practices medicine, diagnosing and treating diseases, injuries, and other physical and mental impairments.