Solved

A College Admissions Counselor Was Interested in Finding Out How

question 22

Essay

A college admissions counselor was interested in finding out how well high school grade point averages (HS GPA) predict first-year college GPAs (FY GPA). A random sample of data from first-year students was reviewed to obtain high school and first-year college GPAs. The data are shown below:

$\begin{array}{|l|l|l|l|l|l|l|l|l|}\hline \text {HS GPA }& 3.82 & 3.90 & 3.20 & 3.40 & 3.88 & 3.50 & 3.60 & 3.70 \\\hline \text {FY GPA} & 3.75 & 3.45 & 2.60 & 2.95 & 3.50 & 2.76 & 3.10 & 3.40 \\\hline\end{array}$

$\begin{array}{|c|c|c|c|c|c|c|c|c|}\hline\text { HS GPA} & 4.00 & 3.30 & 3.50 & 3.80 & 3.87 & 4.00 & 3.20 & 3.82 \\\hline\text { FY GPA }& 3.90 & 2.70 & 3.00 & 3.00 & 3.10 & 3.77 & 2.80 & 3.54 \\\hline\end{array}$

Dependent variable is: $\quad$ FY GPA
No Selector
$\mathrm{R}$ squared $=75.4 \% \quad \mathrm{R}$ squared (adjusted) $=73.6 \%$
$s=0.2118$ with $16-2=14$ degrees of freedom

$\begin{array}{llrrr}\text { Source } & \text { Sum of Squares } & \text { df } & \text { Mean Square } & \text { F-ratio } \\ \text { Regression } & 1.92283 & 1 & 1.92283 & 42.9 \\ \text { Residual } & 0.627867 & 14 & 0.044848 & \\ & & & & \\ \text { Variable } & \text { Coefticient } & \text { s.e. of Coeft } & \text { t-ratio } & \text { prob } \\ \text { Constant } & -1.56410 & 0.7306 & -2.14 & 0.0504 \\ \text { HS GPA } & 1.30527 & 0.1993 & 6.55 & \leq 0.0001\end{array}$

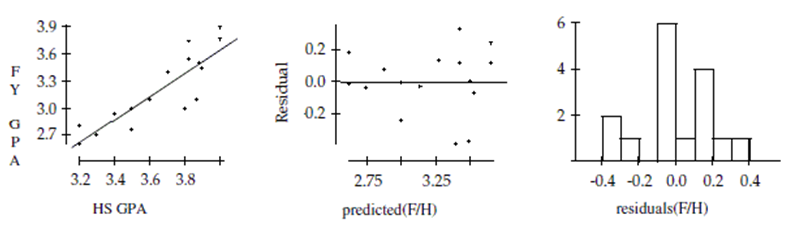
$A college admissions counselor was interested in finding out how well high school grade point averages (HS GPA) predict first-year college GPAs (FY GPA). A random sample of data from first-year students was reviewed to obtain high school and first-year college GPAs. The data are shown below: \begin{array}{|l|l|l|l|l|l|l|l|l|} \hline \text {HS GPA }& 3.82 & 3.90 & 3.20 & 3.40 & 3.88 & 3.50 & 3.60 & 3.70 \\ \hline \text {FY GPA} & 3.75 & 3.45 & 2.60 & 2.95 & 3.50 & 2.76 & 3.10 & 3.40 \\ \hline \end{array} \begin{array}{|c|c|c|c|c|c|c|c|c|} \hline\text { HS GPA} & 4.00 & 3.30 & 3.50 & 3.80 & 3.87 & 4.00 & 3.20 & 3.82 \\ \hline\text { FY GPA }& 3.90 & 2.70 & 3.00 & 3.00 & 3.10 & 3.77 & 2.80 & 3.54 \\ \hline \end{array} Dependent variable is: \quad FY GPA No Selector \mathrm{R} squared =75.4 \% \quad \mathrm{R} squared (adjusted) =73.6 \% s=0.2118 with 16-2=14 degrees of freedom \begin{array}{llrrr}\text { Source } & \text { Sum of Squares } & \text { df } & \text { Mean Square } & \text { F-ratio } \\ \text { Regression } & 1.92283 & 1 & 1.92283 & 42.9 \\ \text { Residual } & 0.627867 & 14 & 0.044848 & \\ & & & & \\ \text { Variable } & \text { Coefticient } & \text { s.e. of Coeft } & \text { t-ratio } & \text { prob } \\ \text { Constant } & -1.56410 & 0.7306 & -2.14 & 0.0504 \\ \text { HS GPA } & 1.30527 & 0.1993 & 6.55 & \leq 0.0001\end{array} -Is there evidence of an association between high school and first-year college GPAs? Test an appropriate hypothesis and state your conclusion in the proper context.$
-Is there evidence of an association between high school and first-year college GPAs? Test an appropriate hypothesis and state your conclusion in the proper context.

Definitions:

Conventional Motivations

Conventional motivations refer to standard or widely accepted reasons that drive people's actions within a society.

Child

A young human being below the age of puberty or below the legal age of majority.

Gay

A term used to describe individuals who are romantically or sexually attracted to people of the same sex.

Parents

Adults who have children or who have the responsibility of raising children, either biological or adopted.