A Statistics Professor Investigated Some of the Factors That Affect $y = \beta _ { 0 } + \beta _ { 1 } x _ { 1 } + \beta _ { 2 } x _ { 2 } + \beta _ { 3 } x _ { 3 } + \varepsilon$

question 50

Essay

A statistics professor investigated some of the factors that affect an individual student's final grade in his or her course. He proposed the multiple regression model: $y = \beta _ { 0 } + \beta _ { 1 } x _ { 1 } + \beta _ { 2 } x _ { 2 } + \beta _ { 3 } x _ { 3 } + \varepsilon$ .
Where:
y = final mark (out of 100). $x _ { 1 }$ = number of lectures skipped. $x _ { 2 }$ = number of late assignments. $x _ { 3 }$ = mid-term test mark (out of 100).
The professor recorded the data for 50 randomly selected students. The computer output is shown below.
THE REGRESSION EQUATION IS 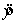= $41.6 - 3.18 x _ { 1 } - 1.17 x _ { 2 } + .63 x _ { 3 }$ $$\begin{array} { | c | c c c | } \hline \text { Predictor } & \text { Coef } & \text { StDev } & \mathrm { T } \\\hline \text { Constant } & 41.6 & 17.8 & 2.337 \\x _ { 1 } & - 3.18 & 1.66 & - 1.916 \\x _ { 2 } & - 1.17 & 1.13 & - 1.035 \\x _ { 3 } & 0.63 & 0.13 & 4.846 \\\hline\end{array}$$ S = 13.74 R-Sq = 30.0%. $$\begin{array}{l}\text { ANALYSIS OF VARIANCE }\\\begin{array} { | l | c c c c | } \hline \text { Source of Variation } & \mathrm { df } & \text { SS } & \text { MS } & \text { F } \\\hline \text { Regression } & 3 & 3716 & 1238.667 & 6.558 \\\text { Error } & 46 & 8688 & 188.870 & \\\hline \text { Total } & 49 & 12404 & & \\\hline\end{array}\end{array}$$ Do these data provide enough evidence at the 1% significance level to conclude that the final mark and the mid-term mark are positively linearly related?

---

Definitions:

Formal Codes

Formal codes are established guidelines or systems of rules, often written, that dictate the expected conduct or procedures within an organization or community.

Ethics

Principals that define right and wrong behavior in conducting one’s personal and professional life.

Workforce Diversity

Describes differences among workers in gender, race, age, ethnic culture, able‐bodiedness, religious affiliation, and sexual orientation.

Ethical Dilemmas

Situations in which a choice must be made between two options, neither of which resolves the situation in an ethically acceptable manner.