SCENARIO 16-13
Given Below Is the Monthly Time Series Data

question 21

Short Answer

SCENARIO 16-13
Given below is the monthly time series data for U.S. retail sales of building materials over a
specific year. $$\begin{array} { | c | c | } \hline \text { Month } & \text { Retail Sales } \\\hline 1 & 6,594 \\\hline 2 & 6,610 \\\hline 3 & 8,174 \\\hline 4 & 9,513 \\\hline 5 & 10,595 \\\hline 6 & 10,415 \\\hline 7 & 9,949 \\\hline 8 & 9,810 \\\hline 9 & 9,637 \\\hline 10 & 9,732 \\\hline 11 & 9,214 \\\hline 12 & 9,201 \\\hline\end{array}$$ The results of the linear trend, quadratic trend, exponential trend, first-order autoregressive,
second-order autoregressive and third-order autoregressive model are presented below in which
the coded month for the 1st month is 0: $\text { Linear trend model: }$
$\begin{array}{lrrrr} & \text { Coefficients } & \text { Standard Error } & \text { t Stat } & \text { P-value } \\\hline \text { Intercept } & 7950.7564 & 617.6342 & 12.8729 & 0.0000 \\\text { Coded Month } & 212.6503 & 95.1145 & 2.2357 & 0.0494\end{array}$

$\text { Quadratic trend model: }$


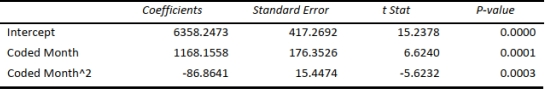
$\text { Exponential trend model: }$
$\begin{array}{lrrrr}\hline & \text { Coefficients } & \text { Standard Error } & \text { t Stat } & \text { P-value } \\\hline \text { Intercept } & 3.8912 & 0.0315 & 123.3674 & 0.0000 \\\text { Coded Month } & 0.0116 & 0.0049 & 2.3957 & 0.0376\end{array}$


$\text { First-order autoregressive: }$
$\begin{array}{lrrrr} & \text { Coefficients } & \text { Standard Error } & t \text { Stat } & {\text { P-value }} \\\hline \text { Intercept } & 3132.0951 & 1287.2899 & 2.4331 & 0.0378 \\\text { YLag1 } & 0.6823 & 0.1398 & 4.8812 & 0.0009 \\\hline\end{array}$


-Referring to Scenario 16-13, what is your forecast for the $13 ^ { \text {th } }$ month using the first-order
autoregressive model?

Understand the relationship between sales and production budgeting.

Calculate ending inventory levels based on sales budget projections.

Prepare a sales budget incorporating inventory considerations.

Understand the importance of maintaining inventory buffer for production components.

---

Definitions:

Divorce Rate

A statistical measure representing the frequency of divorces in a population over a certain period of time, often calculated per 1,000 individuals in the population.

Cohabit

To live together in a shared dwelling, typically referring to a couple who are not legally married.

Traditional Views

Perspectives or beliefs that emphasize values, practices, and norms established and maintained over long historical periods, often resistant to change.

Polygamy

Marriage in which a person has more than one spouse and is permitted sexual access to each of them.