SCENARIO 14-11
a Weight-Loss Clinic Wants to Use Regression Analysis $Y=$

question 91

Short Answer

SCENARIO 14-11
A weight-loss clinic wants to use regression analysis to build a model for weight loss of a client
(measured in pounds). Two variables thought to affect weight loss are client's length of time on the
weight-loss program and time of session. These variables are described below: $Y=$ Weight loss (in pounds)
$X_{1}=$ Length of time in weight-loss program (in months)
$X_{2}=1$ if morning session, 0 if not
Data for 25 clients on a weight-loss program at the clinic were collected and used to fit the interaction model: $Y=\beta_{0}+\beta_{1} X_{1}+\beta_{2} X_{2}+\beta_{3} X_{1} X_{2}+\varepsilon$

$\text { Output from Microsoft Excel follows: }$

$\begin{array}{lr}{\text { Regression Statistics }} \\\hline \text { Multiple R } & 0.7308 \\\text { R Square } & 0.5341 \\\text { Adjusted R Square } & 0.4675 \\\text { Standard Error } & 43.3275 \\\text { Observations } & 25 \\\hline\end{array}$

$\text { ANOVA }$


$\begin{array}{lrrrrrrr}\hline & \text { Coefficients } & \text { Standard Error } & {t \text { Stot }} & \rho_{\text {-value }} & \text { Lower 99\% } & \text { Upper 99\% } \\\hline \text { Intercept } & -20.7298 & 22.3710 & -0.9266 & 0.3646 & -84.0702 & 42.6106 \\\text { Length } & 7.2472 & 1.4992 & 4.8340 & 0.0001 & 3.0024 & 11.4919 \\\text { Morn } & 90.1981 & 40.2336 & 2.2419 & 0.0359 & -23.7176 & 204.1138 \\\text { Length × Morn } & -5.1024 & 3.3511 & -1.5226 & 0.1428 & -14.5905 & 4.3857\end{array}$


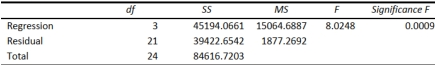
-Referring to Scenario 14-11, which of the following statements is supported by the analysis shown? a) There is sufficient evidence (at $\alpha = 0.05$ ) of curvature in the relationship between weight loss $( Y )$ and months on program $\left( X _ { 1 } \right)$ .
b) There is sufficient evidence (at $\alpha = 0.05$ ) to indicate that the relationship between weight loss $( Y )$ and months on program $\left( X _ { 1 } \right)$ varies with session time.
c) There is insufficient evidence (at $\alpha = 0.05$ ) of curvature in the relationship between weight loss $( Y )$ and months on program $\left( X _ { 1 } \right)$ .
d) There is insufficient evidence (at $\alpha = 0.05$ ) to indicate that the relationship between weight loss $( Y )$ and months on program $\left( X _ { 1 } \right)$ varies with session time.

Recognize the importance of current liabilities and assets and how they are reported.

Understand the concept of the current ratio and its significance in assessing a company’s liquidity.

Acknowledge the role and preparation of adjusting entries in the accounting process.

Comprehend the function and benefits of using a worksheet in the accounting cycle.

---

Definitions:

Overgeneralization

A cognitive distortion where an individual concludes a general rule or pattern from a single incident or a limited set of evidence, often leading to exaggerated conclusions.

Therapeutic Lifestyle

Involves adopting habits and behaviors that promote mental, physical, and emotional health.

Community Mental Health Movement

An approach aimed at improving mental health care access and treatment for individuals within their own communities, emphasizing deinstitutionalization and local support services.

Social System

An organized set of interrelated structures and patterns of behavior that influence individual and group dynamics within a society.