SCENARIO 14-16
What Are the Factors That Determine the Acceleration $Y$

question 321

True/False

SCENARIO 14-16
What are the factors that determine the acceleration time (in sec.) from 0 to 60 miles per hour of a
car? Data on the following variables for 30 different vehicle models were collected: $Y$ (Accel Time): Acceleration time in sec.
$X _ { I }$ (Engine Size): c.c.
$X _ { 2 }$ (Sedan): 1 if the vehicle model is a sedan and 0 otherwise

The regression results using acceleration time as the dependent variable and the remaining variables as the independent variables are presented below.

$\begin{array}{lr}\hline{\text { Regression Statistics }} \\\hline \text { Multiple R } & 0.6096 \\\text { R Square } & 0.3716 \\\text { Adjusted R Square } & 0.3251 \\\text { Standard Error } & 1.4629 \\\text { Observations } & 30 \\\hline\end{array}$

ANOVA



$\begin{array}{lrrrrrr}\hline & \text { Coefficients } & \text { Standard Error } & \text { t Stat } & \text { P-value } & \text { Lower 95\% } & \text { Upper 95\% } \\\hline \text { Intercept } & 7.1052 & 0.6574 & 10.8086 & 0.0000 & 5.7564 & 8.4540 \\\text { Engine Size } & -0.0005 & 0.0001 & -3.6477 & 0.0011 & -0.0008 & -0.0002 \\\text { Sedan } & 0.7264 & 0.5564 & 1.3056 & 0.2027 & -0.4152 & 1.8681 \\\hline\end{array}$


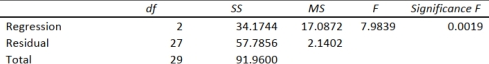
-Referring to Scenario 14-16, there is enough evidence to conclude that engine
size makes a significant contribution to the regression model in the presence of the other
independent variable at a 5% level of significance.

---

Definitions:

Value Increase

An appreciation in the worth or market value of an asset or investment over time.

Intrinsic Value

The perceived or calculated true value of an asset, investment, or company based on fundamental analysis without reference to its market value.

Lower Bound

The minimum value that a mathematical function, financial security, or market variable can have.

Call's Value

Represents the price of an option to buy a particular stock or asset at a set price within a specific time frame.