SCENARIO 14-8 a Financial Analyst Wanted to Examine the Relationship $\$ 1,000$

question 58

Short Answer

SCENARIO 14-8 A financial analyst wanted to examine the relationship between salary (in $\$ 1,000$ ) and 2 variables: age $\left(X_{1}=\mathrm{Age}\right)$ and experience in the field $\left(X_{2}=\right.$ Exper). He took a sample of 20 employees and obtained the following Microsoft Excel output:

$\begin{array}{lr}\hline {\text { Regression Statistics }} \\\hline \text { Multiple R } & 0.8535 \\\text { R Square } & 0.7284 \\\text { Adjusted R Square } & 0.6964 \\\text { Standard Error } & 10.5630 \\\text { Observations } & 20 \\\hline\end{array}$

$\text { ANOVA }$


$\begin{array}{lrrrrrr} & \text { Coefficients } & \text { Standard Error } & {\text { t Stat }} & \text { P-value } & \text { Lower 95\% } &{\text { O5\% }} \\\hline \text { Intercept } & 1.5740 & 9.2723 & 0.1698 & 0.8672 & -17.9888 & 21.1368 \\\text { Age } & 1.3045 & 0.1956 & 6.6678 & 0.0000 & 0.8917 & 1.7173 \\\text { Exper } & -0.1478 & 0.1944 & -0.7604 & 0.4574 & -0.5580 & 0.2624 \\\hline\end{array}$
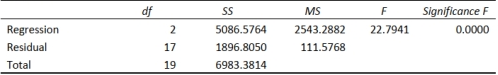
Also the sum of squares due to the regression for the model that includes only Age is 5022.0654 while the
sum of squares due to the regression for the model that includes only Exper is 125.9848.
-Referring to Scenario 14-8, the analyst wants to use a t test to test for the significance of the
coefficient of X2. For a level of significance of 0.01, the critical values of the test are ________.

---

Definitions:

Stroboscopic Motion

The visual perception of motion produced by a rapid succession of static images, as in motion pictures.

Texture Gradients

A visual cue in perception where the texture of objects becomes denser and finer as the distance from the observer increases, aiding in the perception of depth.

Object Constancy

The understanding that objects remain the same, irrespective of changes in viewing conditions such as angle, distance, or lighting.

Size

A dimension that determines how large or small something is.