It Is Possible That the Distance That a City Is $= 87.6 \% \quad$

question 323

Essay

It is possible that the distance that a city is from the ocean could affect its average January
low temperature. Coast gives an approximate distance of each city from the East Coast or
West Coast (whichever is nearer). Including it in the regression yields the following
regression table: Dependent variable is:JanTemp
R squared $= 87.6 \% \quad$ R squared (adjusted) $= 86.9 \%$ $\mathrm { s } = 4.878$ with $55 - 4 = 51$ degrees of freedom
$\begin{array} { l c c c c } \text { Source } & \text { Sum of Squares } & \text { df } & \text { Mean Square } & \text { F-ratio } \\ \text { Regression } 8611.86 & 3 & 2870.62 & 121 \\ \text { Residual } & 1213.67 & 51 & 23.7974 & \end{array}\\$
$\begin{array} { l c l l r } \text { Variable } & \text { Coefficient } & \text { SE(Coeff) } & \text { t-ratio } & \text { P-value } \\ \text { Intercept } & 111.878 & 6.167 & 18.1 & \leq 0.0001 \\ \text { Lat } & - 2.47722 & 0.1307 & - 19.0 & \leq 0.0001 \\ \text { Long } & 0.221997 & 0.0462 & 4.81 & \leq 0.0001 \\ \text { Coast } & - 0.674929 & 0.0901 & - 7.49 & \leq 0.0001 \end{array}$
And here is a scatterplot of the residuals: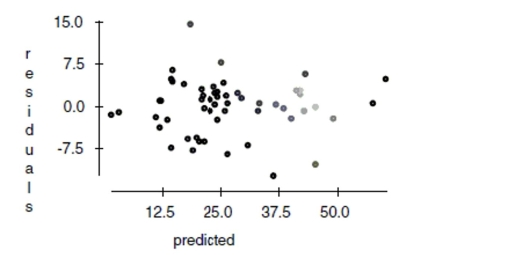
$$\text { Write a report on this regression. Interpret the coefficients and } R ^ { 2 } \text {. Are the conditions met? }$$

---

Definitions:

Customer Cost Analysis

The process of identifying all the costs associated with acquiring and maintaining customers to assess profitability.

Time-Driven Activity-Based Costing

A costing method that calculates costs based on the actual time activities take and the cost of supplying resources to these activities.

Customer Support Department

A specialized team within a company tasked with addressing customer inquiries, problems, and needs, aiming to enhance customer satisfaction and loyalty.

Customer Cost Analysis

An evaluation of all the costs involved in acquiring and retaining customers, used to determine the profitability of customer relationships.