Answer the Question $f ( x , y )$ (Thin Curves) as Well as the Constraint

question 227

Multiple Choice

Answer the question.
-The graph below shows the level curves of a differentiable function $f ( x , y )$ (thin curves) as well as the constraint $g ( x , y ) = \sqrt { x ^ { 2 } + y ^ { 2 } } - \frac { 3 } { 2 } = 0$ (thick circle) . Using the concepts of the orthogonal gradient theorem and the method of Lagrange multipliers, estimate the coordinates corresponding to the constrained extrema of 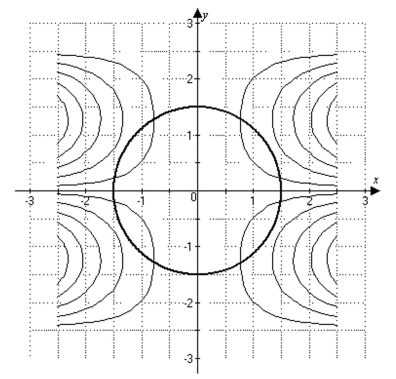$f ( x , y )$ .

• A) (1.3, 0.7), (-1.3, 0.7), (-1.3,-0.7), (1.3,-0.7)
• B) (1.5, 0), (0, 1.5), (-1.5, 0), (0, -1.5)
• C) (1.1, 1.1), (-1.1, 1.1), (-1.1,-1.1), (1.1,-1.1)
• D) (1.5, 0.2), (0.7, 1.3), (-1.5, 0.2), (-0.7, 1.3), (-1.5, -0.2), (-0.7, -1.3), (1.5, -0.2), (0.7, -1.3)

---

Definitions:

X-rays

Electromagnetic waves with wavelengths shorter than those of visible light, capable of penetrating solids and ionizing gases, widely used in medicine and industry for imaging and diagnostic purposes.

Bones

Hard, dense connective tissue forming the skeleton, providing structure and protection to the body as well as assisting with movement.

Dense

Characteristic of a substance having closely packed particles or elements, indicating high mass per unit volume.

X-ray Imaging

A diagnostic technique that uses X-rays, a form of electromagnetic radiation, to create images of the inside of the body, which can be used to detect fractures, infections, and other abnormalities.