Determine Whether the Mean Value Theorem Can Be Applied to the Function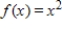

question 69

Multiple Choice

Determine whether the Mean Value Theorem can be applied to the function on the closed interval 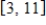. If the Mean Value Theorem can be applied, find all numbers c in the open interval 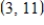such that . ​

• A) MVT applies;
• B) MVT applies;
• C) MVT applies;
• D) MVT applies;
• E) MVT applies;

---

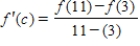Definitions:

Distribution

In statistics, the way in which data or values are spread or distributed across possible values or intervals.

Normal Distribution

A symmetric, bell-shaped distribution of data where most of the observations cluster around the central peak and the probabilities for values further away from the mean taper off equally in both directions.

Standard Deviations

A measure of the amount of variation or dispersion in a set of values, indicating how spread out the data points are from the mean.

Deviation

The amount by which a single measurement differs from a fixed value such as the mean.