TABLE 13-15
The superintendent of a school district wanted to predict the percentage of students passing a sixth-grade proficiency test. She obtained the data on percentage of students passing the proficiency test (% Passing) , daily mean of the percentage of students attending class (% Attendance) , mean teacher salary in dollars (Salaries) , and instructional spending per pupil in dollars (Spending) of 47 schools in the state.
Following is the multiple regression output with Y = % Passing as the dependent variable,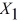 
= : % Attendance, 
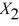= Salaries and 
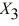= Spending:
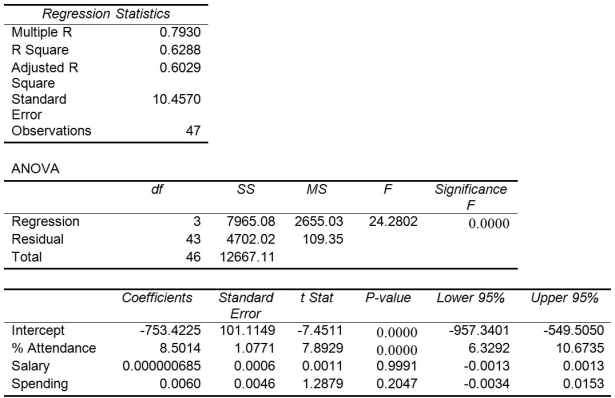
-Referring to Table 13-15, which of the following is a correct statement?
Definitions:
Spoken Language
A socially acquired system of sound patterns with meanings agreed on by the members of a group.
Basic Components
Fundamental elements or parts that make up a whole system, structure, or concept.
Feedback
Information about reactions to a product, a person's performance of a task, etc., used as a basis for improvement.
Deficit Theories
Theories that attribute lower educational performance to cultural, social, linguistic, or intellectual deficits in certain groups.
Q17: When testing for independence in a contingency
Q17: Employment _ occurs when an employee's agreement
Q22: Referring to Table 11-6, the null hypothesis
Q39: _ bonuses are awarded to executives by
Q39: What are three challenges employers face when
Q55: Referring to Table 13-15, the null hypothesis
Q86: Referring to Table 14-5, a p control
Q87: Referring to Table 12-3, the director of
Q101: Referring to Table 12-10, the p-value of
Q160: Referring to Table 13-14, the predicted mileage