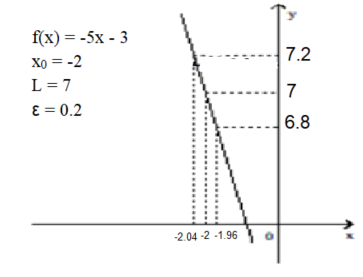
Find the Limit $L$ For the Given Function $f$ , the Point $x _ { 0 }$

question 203

Multiple Choice

Find the limit $L$ for the given function $f$ , the point $x _ { 0 }$ , and the positive number $\varepsilon$ . Then find a number $\delta > 0$ such that, for all $x _ { t } 0 < \left| x - x _ { 0 } \right| < \delta \Rightarrow | f ( x ) - L | < \varepsilon$ .
-

• A) 15
• B) 0.4
• C) 0.04
• D) -0.04

---

Definitions:

Marketing Strategies

Comprehensive plans formulated to achieve specific marketing goals, such as increasing market share, promoting brand awareness, or launching new products.

Pro-Forma Profit-And-Loss Statement

A projected financial statement that estimates a company's future profits or losses based on forecasted revenues and expenses.

Initial Capital Requirements

The amount of funding a business needs to start its operations, covering costs such as inventory, leasing, and other initial expenses.

Financial Ratios

Quantitative measures derived from financial statement analysis used to evaluate a company's financial health, performance, and operational efficiency.