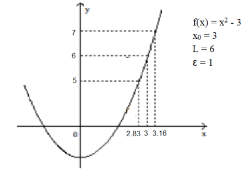
Find the Limit $L$ For the Given Function $f$ , the Point $x _ { 0 }$

question 50

Multiple Choice

Find the limit $L$ for the given function $f$ , the point $x _ { 0 }$ , and the positive number $\varepsilon$ . Then find a number $\delta > 0$ such that, for all $x _ { t } 0 < \left| x - x _ { 0 } \right| < \delta \Rightarrow | f ( x ) - L | < \varepsilon$ .
-

• A) 0.17
• B) 3
• C) 0.33
• D) 0.16

---

Definitions:

Syndesmoses

A type of fibrous joint in which bones are joined together by a ligament or a fibrous tissue, allowing for minimal movement.

Gomphoses

A type of fibrous joint that is a peg-in-socket, such as the joint between a tooth and its socket in the jaw.

Hip And Shoulder

Joints that connect the limbs to the torso; the hip being the articulation between the pelvis and the femur, and the shoulder connecting the upper arm to the torso.

Gliding Motion

A smooth movement where surfaces slide past one another, typical in some types of joints like the carpals of the wrist.