SCENARIO 15-4 the Superintendent of a School District Wanted to Predict

question 17

Multiple Choice

SCENARIO 15-4 The superintendent of a school district wanted to predict the percentage of students passing a sixth-grade proficiency test.She obtained the data on percentage of students passing the proficiency test (% Passing) , daily mean of the percentage of students attending class (% Attendance) , mean teacher salary in dollars (Salaries) , and instructional spending per pupil in dollars (Spending) of 47 schools in the state. Let Y = % Passing as the dependent variable, 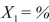Attendance, 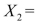Salaries and 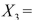Spending. The coefficient of multiple determination ( 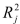) of each of the 3 predictors with all the other remaining predictors are, respectively, 0.0338, 0.4669, and 0.4743. The output from the best-subset regressions is given below: 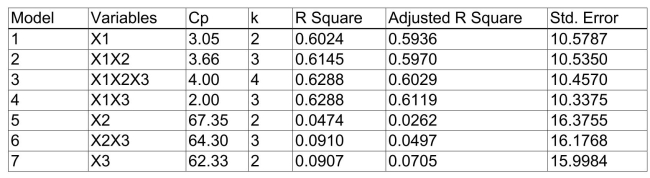Following is the residual plot for % Attendance: 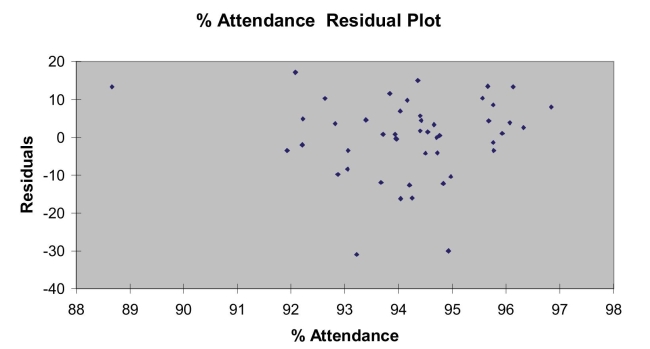Following is the output of several multiple regression models: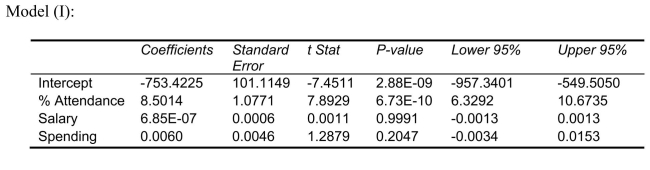
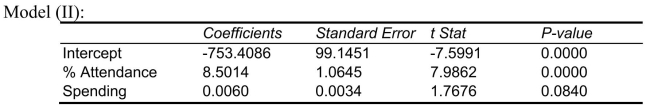
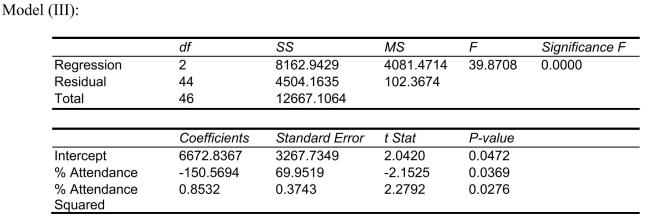
-Referring to Scenario 15-4, which of the following predictors should first be dropped to remove collinearity?

---

Definitions:

Lateral Geniculate Nucleus

A structure in the brain that is part of the thalamus; it acts as a primary processing center for visual information received from the retina.

Cones

Photoreceptor cells in the retina of the eye responsible for color vision and functioning best in relatively bright light.

Retinal Ganglion Cells

Retinal ganglion cells are neurons located in the retina that transmit visual information from the eye to the brain through their axons, which form the optic nerve.

Partial Lack

A situation where there is a deficiency or insufficiency of something, but it is not completely absent.