Willy's Only Source of Wealth Is His Chocolate Factory

question 33

Multiple Choice

Willy's only source of wealth is his chocolate factory. He has the utility function pc 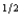_f + (1 - p) c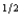
_nf, where p is the probability of a flood, 1 - p is the probability of no flood, and c_f and c_nf are his wealth contingent on a flood and on no flood, respectively. The probability of a flood is p = 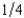. The value of Willy's factory is $300,000 if there is no flood and $0 if there is a flood. Willy can buy insurance where if he buys $x worth of insurance, he must pay the insurance company 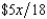whether there is a flood or not but he gets back $x from the company if there is a flood. Willy should buy

• A) enough insurance so that if there is a flood, after he collects his insurance, his wealth will beof what it would be if there were no flood.
• B) enough insurance so that if there is a flood, after he collects his insurance, his wealth will be the same whether there was a flood or not.
• C) no insurance since the cost per dollar of insurance exceeds the probability of a flood.
• D) .enough insurance so that if there is a flood, after he collects his insurance, his wealth will beof what it would be if there were no flood.
• E) enough insurance so that if there is a flood, after he collects his insurance his wealth will beof what it would be if there were no flood

---

Definitions:

Noncompete Clause

A provision often included in a contract to purchase a business that restricts the seller from entering the same type of business within a specified area for a certain amount of time.

Intellectual Property Contract

A legal agreement that outlines the terms of use, distribution, and ownership of intellectual property between two parties.

Similar Business

A company that operates in the same industry or offers similar products or services as another business.

Last Minute

Refers to actions or decisions made very close to a deadline or an event's scheduled time.