Examlex

Solved

The Following Table Shows the Annual Revenues (In Millions of Dollars)

question 16

Essay

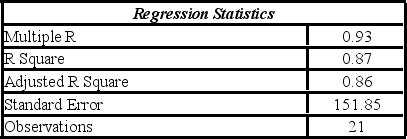
The following table shows the annual revenues (in millions of dollars) of a pharmaceutical company over the period 1990-2011. The following table shows the annual revenues (in millions of dollars) of a pharmaceutical company over the period 1990-2011. The autoregressive models of order 1 and 2, y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + ε<sub>t</sub>, and y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + β<sub>2</sub>y<sub>t - 2</sub> + ε<sub>t</sub>, were applied on the time series to make revenue forecasts. The relevant parts of Excel regression outputs are given below. Model AR(1): Model AR(2): When for AR(1), H<sub>0</sub>: β<sub>0</sub> = 0 is tested against H<sub>A</sub>: β<sub>0</sub> ≠ 0, the p-value of this t test shown the output as 0.9590. This could suggest that the model y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1 </sub>+ ε<sub>t</sub> might be an alternative to the AR(1) model y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t-1</sub> + ε<sub>t</sub>. Partial output for this simplified model is as follows: Find the revenue forecast for 2012 through the use of y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1</sub> + ε<sub>t</sub>. The autoregressive models of order 1 and 2, yt = β0 + β1yt - 1 + εt, and yt = β0 + β1yt - 1 + β2yt - 2 + εt, were applied on the time series to make revenue forecasts. The relevant parts of Excel regression outputs are given below.
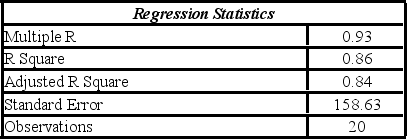
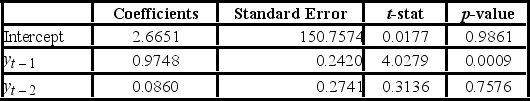Model AR(1): The following table shows the annual revenues (in millions of dollars) of a pharmaceutical company over the period 1990-2011. The autoregressive models of order 1 and 2, y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + ε<sub>t</sub>, and y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + β<sub>2</sub>y<sub>t - 2</sub> + ε<sub>t</sub>, were applied on the time series to make revenue forecasts. The relevant parts of Excel regression outputs are given below. Model AR(1): Model AR(2): When for AR(1), H<sub>0</sub>: β<sub>0</sub> = 0 is tested against H<sub>A</sub>: β<sub>0</sub> ≠ 0, the p-value of this t test shown the output as 0.9590. This could suggest that the model y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1 </sub>+ ε<sub>t</sub> might be an alternative to the AR(1) model y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t-1</sub> + ε<sub>t</sub>. Partial output for this simplified model is as follows: Find the revenue forecast for 2012 through the use of y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1</sub> + ε<sub>t</sub>. The following table shows the annual revenues (in millions of dollars) of a pharmaceutical company over the period 1990-2011. The autoregressive models of order 1 and 2, y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + ε<sub>t</sub>, and y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + β<sub>2</sub>y<sub>t - 2</sub> + ε<sub>t</sub>, were applied on the time series to make revenue forecasts. The relevant parts of Excel regression outputs are given below. Model AR(1): Model AR(2): When for AR(1), H<sub>0</sub>: β<sub>0</sub> = 0 is tested against H<sub>A</sub>: β<sub>0</sub> ≠ 0, the p-value of this t test shown the output as 0.9590. This could suggest that the model y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1 </sub>+ ε<sub>t</sub> might be an alternative to the AR(1) model y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t-1</sub> + ε<sub>t</sub>. Partial output for this simplified model is as follows: Find the revenue forecast for 2012 through the use of y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1</sub> + ε<sub>t</sub>. Model AR(2): The following table shows the annual revenues (in millions of dollars) of a pharmaceutical company over the period 1990-2011. The autoregressive models of order 1 and 2, y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + ε<sub>t</sub>, and y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + β<sub>2</sub>y<sub>t - 2</sub> + ε<sub>t</sub>, were applied on the time series to make revenue forecasts. The relevant parts of Excel regression outputs are given below. Model AR(1): Model AR(2): When for AR(1), H<sub>0</sub>: β<sub>0</sub> = 0 is tested against H<sub>A</sub>: β<sub>0</sub> ≠ 0, the p-value of this t test shown the output as 0.9590. This could suggest that the model y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1 </sub>+ ε<sub>t</sub> might be an alternative to the AR(1) model y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t-1</sub> + ε<sub>t</sub>. Partial output for this simplified model is as follows: Find the revenue forecast for 2012 through the use of y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1</sub> + ε<sub>t</sub>. The following table shows the annual revenues (in millions of dollars) of a pharmaceutical company over the period 1990-2011. The autoregressive models of order 1 and 2, y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + ε<sub>t</sub>, and y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + β<sub>2</sub>y<sub>t - 2</sub> + ε<sub>t</sub>, were applied on the time series to make revenue forecasts. The relevant parts of Excel regression outputs are given below. Model AR(1): Model AR(2): When for AR(1), H<sub>0</sub>: β<sub>0</sub> = 0 is tested against H<sub>A</sub>: β<sub>0</sub> ≠ 0, the p-value of this t test shown the output as 0.9590. This could suggest that the model y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1 </sub>+ ε<sub>t</sub> might be an alternative to the AR(1) model y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t-1</sub> + ε<sub>t</sub>. Partial output for this simplified model is as follows: Find the revenue forecast for 2012 through the use of y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1</sub> + ε<sub>t</sub>. When for AR(1), H0: β0 = 0 is tested against HA: β0 ≠ 0, the p-value of this t test shown the output as 0.9590. This could suggest that the model yt = β1yt-1 + εt might be an alternative to the AR(1) model yt = β0 + β1yt-1 + εt. Partial output for this simplified model is as follows: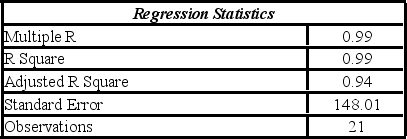 The following table shows the annual revenues (in millions of dollars) of a pharmaceutical company over the period 1990-2011. The autoregressive models of order 1 and 2, y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + ε<sub>t</sub>, and y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + β<sub>2</sub>y<sub>t - 2</sub> + ε<sub>t</sub>, were applied on the time series to make revenue forecasts. The relevant parts of Excel regression outputs are given below. Model AR(1): Model AR(2): When for AR(1), H<sub>0</sub>: β<sub>0</sub> = 0 is tested against H<sub>A</sub>: β<sub>0</sub> ≠ 0, the p-value of this t test shown the output as 0.9590. This could suggest that the model y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1 </sub>+ ε<sub>t</sub> might be an alternative to the AR(1) model y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t-1</sub> + ε<sub>t</sub>. Partial output for this simplified model is as follows: Find the revenue forecast for 2012 through the use of y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1</sub> + ε<sub>t</sub>. The following table shows the annual revenues (in millions of dollars) of a pharmaceutical company over the period 1990-2011. The autoregressive models of order 1 and 2, y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + ε<sub>t</sub>, and y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + β<sub>2</sub>y<sub>t - 2</sub> + ε<sub>t</sub>, were applied on the time series to make revenue forecasts. The relevant parts of Excel regression outputs are given below. Model AR(1): Model AR(2): When for AR(1), H<sub>0</sub>: β<sub>0</sub> = 0 is tested against H<sub>A</sub>: β<sub>0</sub> ≠ 0, the p-value of this t test shown the output as 0.9590. This could suggest that the model y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1 </sub>+ ε<sub>t</sub> might be an alternative to the AR(1) model y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t-1</sub> + ε<sub>t</sub>. Partial output for this simplified model is as follows: Find the revenue forecast for 2012 through the use of y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1</sub> + ε<sub>t</sub>. Find the revenue forecast for 2012 through the use of yt = β1yt-1 + εt.

Definitions:

Consolidated Income

The total net income of a parent company and its subsidiaries, after accounting for the share of income pertaining to noncontrolling interests.

Subsidiaries

Companies that are owned or controlled by another company, known as the parent company, through a majority of the voting stock.

Domestic Firm

A company that operates primarily within the borders of its home country and is subject to its home country's jurisdiction and regulations.

Ownership Interest

Indicates an individual's or entity's legal rights and stakes in a company or property.

Related Questions

Q1: Which of the following is a causal

Q23: In which of the following models does

Q47: The nonparametric test for two population medians

Q59: A polynomial trend model that only allows

Q69: Given an inverse yield curve, would the

Q73: Futures contracts have non-negotiable features.

Q102: Firms A, B, and C operate in

Q114: Which of the following factors refers to

Q114: Firms A, B, and C operate in

Q115: A study was conducted to determine if