Examlex

Solved

The Following Table Shows the Annual Revenues (In Millions of Dollars)

question 101

Essay

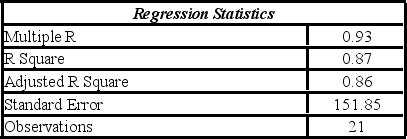
The following table shows the annual revenues (in millions of dollars) of a pharmaceutical company over the period 1990-2011. The following table shows the annual revenues (in millions of dollars) of a pharmaceutical company over the period 1990-2011. The autoregressive models of order 1 and 2, y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + ε<sub>t</sub>, and y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + β<sub>2</sub>y<sub>t - 2</sub> + ε<sub>t</sub>, were applied on the time series to make revenue forecasts. The relevant parts of Excel regression outputs are given below. Model AR(1): Model AR(2): When for AR(1), H<sub>0</sub>: β<sub>0</sub> = 0 is tested against H<sub>A</sub>: β<sub>0</sub> ≠ 0, the p-value of this t test shown by the output as 0.9590. This could suggest that the model y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1 </sub>+ ε<sub>t</sub> might be an alternative to the AR(1) model y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t</sub><sub>-1</sub> + ε<sub>t</sub>. Excel partial output for this simplified model is as follows. Compare the autoregressive models y<sub>t</sub> = β<sub>0 </sub>+ β<sub>1</sub>y<sub>t-1</sub> + ε<sub>t</sub>; y<sub>t</sub> = β<sub>0 </sub>+ β<sub>1</sub>y<sub>t</sub><sub>-1</sub> + β<sub>2</sub>y<sub>t</sub><sub>-2 </sub>+ ε<sub>t</sub>, and y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1</sub> + ε<sub>t</sub>, through the use of MSE and MAD. The autoregressive models of order 1 and 2, yt = β0 + β1yt - 1 + εt, and yt = β0 + β1yt - 1 + β2yt - 2 + εt, were applied on the time series to make revenue forecasts. The relevant parts of Excel regression outputs are given below.
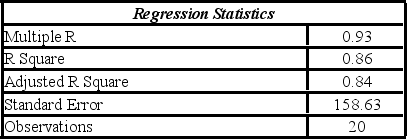
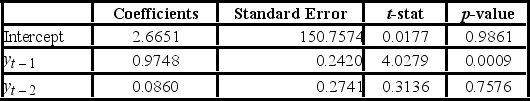Model AR(1): The following table shows the annual revenues (in millions of dollars) of a pharmaceutical company over the period 1990-2011. The autoregressive models of order 1 and 2, y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + ε<sub>t</sub>, and y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + β<sub>2</sub>y<sub>t - 2</sub> + ε<sub>t</sub>, were applied on the time series to make revenue forecasts. The relevant parts of Excel regression outputs are given below. Model AR(1): Model AR(2): When for AR(1), H<sub>0</sub>: β<sub>0</sub> = 0 is tested against H<sub>A</sub>: β<sub>0</sub> ≠ 0, the p-value of this t test shown by the output as 0.9590. This could suggest that the model y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1 </sub>+ ε<sub>t</sub> might be an alternative to the AR(1) model y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t</sub><sub>-1</sub> + ε<sub>t</sub>. Excel partial output for this simplified model is as follows. Compare the autoregressive models y<sub>t</sub> = β<sub>0 </sub>+ β<sub>1</sub>y<sub>t-1</sub> + ε<sub>t</sub>; y<sub>t</sub> = β<sub>0 </sub>+ β<sub>1</sub>y<sub>t</sub><sub>-1</sub> + β<sub>2</sub>y<sub>t</sub><sub>-2 </sub>+ ε<sub>t</sub>, and y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1</sub> + ε<sub>t</sub>, through the use of MSE and MAD. The following table shows the annual revenues (in millions of dollars) of a pharmaceutical company over the period 1990-2011. The autoregressive models of order 1 and 2, y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + ε<sub>t</sub>, and y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + β<sub>2</sub>y<sub>t - 2</sub> + ε<sub>t</sub>, were applied on the time series to make revenue forecasts. The relevant parts of Excel regression outputs are given below. Model AR(1): Model AR(2): When for AR(1), H<sub>0</sub>: β<sub>0</sub> = 0 is tested against H<sub>A</sub>: β<sub>0</sub> ≠ 0, the p-value of this t test shown by the output as 0.9590. This could suggest that the model y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1 </sub>+ ε<sub>t</sub> might be an alternative to the AR(1) model y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t</sub><sub>-1</sub> + ε<sub>t</sub>. Excel partial output for this simplified model is as follows. Compare the autoregressive models y<sub>t</sub> = β<sub>0 </sub>+ β<sub>1</sub>y<sub>t-1</sub> + ε<sub>t</sub>; y<sub>t</sub> = β<sub>0 </sub>+ β<sub>1</sub>y<sub>t</sub><sub>-1</sub> + β<sub>2</sub>y<sub>t</sub><sub>-2 </sub>+ ε<sub>t</sub>, and y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1</sub> + ε<sub>t</sub>, through the use of MSE and MAD. Model AR(2): The following table shows the annual revenues (in millions of dollars) of a pharmaceutical company over the period 1990-2011. The autoregressive models of order 1 and 2, y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + ε<sub>t</sub>, and y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + β<sub>2</sub>y<sub>t - 2</sub> + ε<sub>t</sub>, were applied on the time series to make revenue forecasts. The relevant parts of Excel regression outputs are given below. Model AR(1): Model AR(2): When for AR(1), H<sub>0</sub>: β<sub>0</sub> = 0 is tested against H<sub>A</sub>: β<sub>0</sub> ≠ 0, the p-value of this t test shown by the output as 0.9590. This could suggest that the model y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1 </sub>+ ε<sub>t</sub> might be an alternative to the AR(1) model y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t</sub><sub>-1</sub> + ε<sub>t</sub>. Excel partial output for this simplified model is as follows. Compare the autoregressive models y<sub>t</sub> = β<sub>0 </sub>+ β<sub>1</sub>y<sub>t-1</sub> + ε<sub>t</sub>; y<sub>t</sub> = β<sub>0 </sub>+ β<sub>1</sub>y<sub>t</sub><sub>-1</sub> + β<sub>2</sub>y<sub>t</sub><sub>-2 </sub>+ ε<sub>t</sub>, and y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1</sub> + ε<sub>t</sub>, through the use of MSE and MAD. The following table shows the annual revenues (in millions of dollars) of a pharmaceutical company over the period 1990-2011. The autoregressive models of order 1 and 2, y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + ε<sub>t</sub>, and y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + β<sub>2</sub>y<sub>t - 2</sub> + ε<sub>t</sub>, were applied on the time series to make revenue forecasts. The relevant parts of Excel regression outputs are given below. Model AR(1): Model AR(2): When for AR(1), H<sub>0</sub>: β<sub>0</sub> = 0 is tested against H<sub>A</sub>: β<sub>0</sub> ≠ 0, the p-value of this t test shown by the output as 0.9590. This could suggest that the model y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1 </sub>+ ε<sub>t</sub> might be an alternative to the AR(1) model y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t</sub><sub>-1</sub> + ε<sub>t</sub>. Excel partial output for this simplified model is as follows. Compare the autoregressive models y<sub>t</sub> = β<sub>0 </sub>+ β<sub>1</sub>y<sub>t-1</sub> + ε<sub>t</sub>; y<sub>t</sub> = β<sub>0 </sub>+ β<sub>1</sub>y<sub>t</sub><sub>-1</sub> + β<sub>2</sub>y<sub>t</sub><sub>-2 </sub>+ ε<sub>t</sub>, and y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1</sub> + ε<sub>t</sub>, through the use of MSE and MAD. When for AR(1), H0: β0 = 0 is tested against HA: β0 ≠ 0, the p-value of this t test shown by the output as 0.9590. This could suggest that the model yt = β1yt-1 + εt might be an alternative to the AR(1) model yt = β0 + β1yt-1 + εt. Excel partial output for this simplified model is as follows.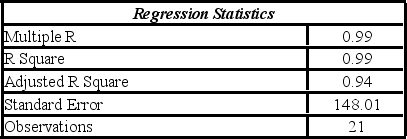 The following table shows the annual revenues (in millions of dollars) of a pharmaceutical company over the period 1990-2011. The autoregressive models of order 1 and 2, y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + ε<sub>t</sub>, and y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + β<sub>2</sub>y<sub>t - 2</sub> + ε<sub>t</sub>, were applied on the time series to make revenue forecasts. The relevant parts of Excel regression outputs are given below. Model AR(1): Model AR(2): When for AR(1), H<sub>0</sub>: β<sub>0</sub> = 0 is tested against H<sub>A</sub>: β<sub>0</sub> ≠ 0, the p-value of this t test shown by the output as 0.9590. This could suggest that the model y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1 </sub>+ ε<sub>t</sub> might be an alternative to the AR(1) model y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t</sub><sub>-1</sub> + ε<sub>t</sub>. Excel partial output for this simplified model is as follows. Compare the autoregressive models y<sub>t</sub> = β<sub>0 </sub>+ β<sub>1</sub>y<sub>t-1</sub> + ε<sub>t</sub>; y<sub>t</sub> = β<sub>0 </sub>+ β<sub>1</sub>y<sub>t</sub><sub>-1</sub> + β<sub>2</sub>y<sub>t</sub><sub>-2 </sub>+ ε<sub>t</sub>, and y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1</sub> + ε<sub>t</sub>, through the use of MSE and MAD. The following table shows the annual revenues (in millions of dollars) of a pharmaceutical company over the period 1990-2011. The autoregressive models of order 1 and 2, y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + ε<sub>t</sub>, and y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + β<sub>2</sub>y<sub>t - 2</sub> + ε<sub>t</sub>, were applied on the time series to make revenue forecasts. The relevant parts of Excel regression outputs are given below. Model AR(1): Model AR(2): When for AR(1), H<sub>0</sub>: β<sub>0</sub> = 0 is tested against H<sub>A</sub>: β<sub>0</sub> ≠ 0, the p-value of this t test shown by the output as 0.9590. This could suggest that the model y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1 </sub>+ ε<sub>t</sub> might be an alternative to the AR(1) model y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t</sub><sub>-1</sub> + ε<sub>t</sub>. Excel partial output for this simplified model is as follows. Compare the autoregressive models y<sub>t</sub> = β<sub>0 </sub>+ β<sub>1</sub>y<sub>t-1</sub> + ε<sub>t</sub>; y<sub>t</sub> = β<sub>0 </sub>+ β<sub>1</sub>y<sub>t</sub><sub>-1</sub> + β<sub>2</sub>y<sub>t</sub><sub>-2 </sub>+ ε<sub>t</sub>, and y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1</sub> + ε<sub>t</sub>, through the use of MSE and MAD. Compare the autoregressive models yt = β0 + β1yt-1 + εt; yt = β0 + β1yt-1 + β2yt-2 + εt, and yt = β1yt-1 + εt, through the use of MSE and MAD.

Definitions:

Anomic Suicide

A concept in sociology referring to a form of suicide that occurs when an individual feels disoriented and disconnected from societal norms and values.

Imitative Suicide

A suicide act that follows or is inspired by another person's suicide, often publicized in the media.

Sociocultural View

A perspective in psychology that emphasizes the influence of cultural and social factors on an individual's behavior and mental processes.

Interpersonal Theory

A psychological framework that explains how the behavior and mental states of individuals are influenced by their relationships and interactions with others.

Related Questions

Q3: Thirty employed single individuals were randomly selected

Q10: Initial margins for various types of futures

Q32: The mean of the absolute residuals defines

Q43: Which of the following is NOT true

Q44: A wine magazine wants to know if

Q55: Since a put is the right to

Q61: The following data, with the corresponding Excel

Q78: In the following diagram: <img src="https://d2lvgg3v3hfg70.cloudfront.net/TB4219/.jpg" alt="In

Q105: Like any other university, Seton Hall University

Q111: Consider the following simple linear regression model: