Instruction 12 $\text { Regression statistics }$ $\text { ANOVA }$

question 77

Multiple Choice

Instruction 12.10
A computer software developer would like to use the number of downloads (in thousands) for the trial version of his new shareware to predict the amount of revenue (in thousands of dollars) he can make on the full version of the new shareware. Following is the output from a simple linear regression along with the residual plot and normal probability plot obtained from a data set of 30 different sharewares that he has developed:
$\text { Regression statistics }$
$\begin{array}{|l|l|}\hline \text { MultipleR } & 0.8691 \\\hline \text { R Square } & 0.7554 \\\hline \begin{array}{l}\text { Adjusted R } \\\text { Square }\end{array} & 0.7467 \\\hline \text { Standard Error } & 44.4765 \\\hline \text { Observations } & 30.0000\\\hline \end{array}$

$\text { ANOVA }$
$\begin{array}{|l|l|l|l|l|l|}\hline & d f & S S & M S & F & \begin{array}{l}\text { Significance } \\F\end{array} \\\hline \text { Regression } & 1 & 171062.9193 & 171062.9193 & 86.4759 & 0.0000 \\\hline \text { Residual } & 28 & 55388.4309 & 1978.1582 & & \\\hline \text { Total } & 29 & 226451.3503 & & & \\\hline\end{array}$

$\begin{array}{|l|l|l|l|l|l|l|}\hline & \text { Coefficients } & \begin{array}{l}\text { Standard } \\\text { Error }\end{array} & \text { t Stat } & p \text {-value } & \text { Lower 95\% } & \text { Upper 95\% } \\\hline \text { Intercept } & -95.0614 & 26.9183 & -3.5315 & 0.0015 & -150.2009 & -39.9218 \\\hline \text { Download } & 3.7297 & 0.4011 & 9.2992 & 0.0000 & 2.9082 & 4.5513 \\\hline\end{array}$
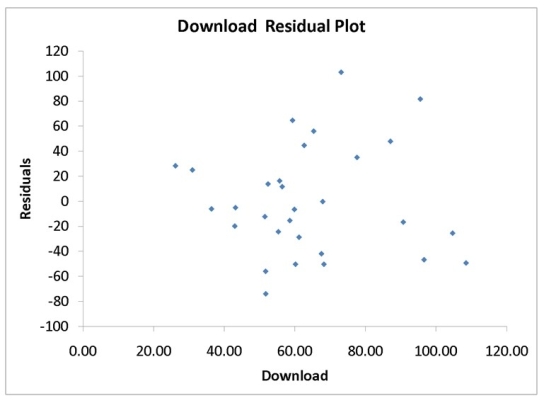
-Referring to Instruction 12.10,which of the following is the correct interpretation for the slope coefficient?

• A) For each increase of 1,000 downloads, the expected revenue is estimated to increase by $3.7297 thousands.
• B) For each increase of 1,000 dollars in expected revenue, the expected number of downloads is estimated to increase by 3.7297 thousands.
• C) For each decrease of 1,000 dollars in expected revenue, the expected number of downloads is estimated to increase by 3.7297 thousands.
• D) For each decrease of 1,000 downloads, the expected revenue is estimated to increase by $3.7297 thousands.

---

Definitions:

Observational Learning

The process of learning behaviors by watching and imitating others.

Positive Reinforcement

A process in behavior analysis that involves the addition of a stimulus following a behavior that makes the behavior more likely to occur again in the future.

Autonomic Arousal

The activation of the autonomic nervous system, which can increase heart rate, blood pressure, and respiration rate, often as a response to stress or excitement.

Schachter and Singer

Psychologists who developed the two-factor theory of emotion, suggesting that emotion is based on physiological arousal and cognitive label.