Instruction 12 $\text { Regression statistics }$ $\text { ANOVA }$

question 36

Multiple Choice

Instruction 12.19
A computer software developer would like to use the number of downloads (in thousands) for the trial version of his new shareware to predict the amount of revenue (in thousands of dollars) he can make on the full version of the new shareware. Following is the output from a simple linear regression along with the residual plot and normal probability plot obtained from a data set of 30 different sharewares that he has developed:
$\text { Regression statistics }$
$\begin{array}{|l|l|}\hline \text { MultipleR } & 0.8691 \\\hline \text { R Square } & 0.7554 \\\hline \begin{array}{l}\text { Adjusted R } \\\text { Square }\end{array} & 0.7467 \\\hline \text { Standard Error } & 44.4765 \\\hline \text { Observations } & 30.0000\\\hline \end{array}$

$\text { ANOVA }$
$\begin{array}{|l|l|l|l|l|l|}\hline & d f & S S & M S & F & \begin{array}{l}\text { Significance } \\F\end{array} \\\hline \text { Regression } & 1 & 171062.9193 & 171062.9193 & 86.4759 & 0.0000 \\\hline \text { Residual } & 28 & 55388.4309 & 1978.1582 & & \\\hline \text { Total } & 29 & 226451.3503 & & & \\\hline\end{array}$

$\begin{array}{|l|l|l|l|l|l|l|}\hline & \text { Coefficients } & \begin{array}{l}\text { Standard } \\\text { Error }\end{array} & \text { t Stat } & p \text {-value } & \text { Lower 95\% } & \text { Upper 95\% } \\\hline \text { Intercept } & -95.0614 & 26.9183 & -3.5315 & 0.0015 & -150.2009 & -39.9218 \\\hline \text { Download } & 3.7297 & 0.4011 & 9.2992 & 0.0000 & 2.9082 & 4.5513 \\\hline\end{array}$


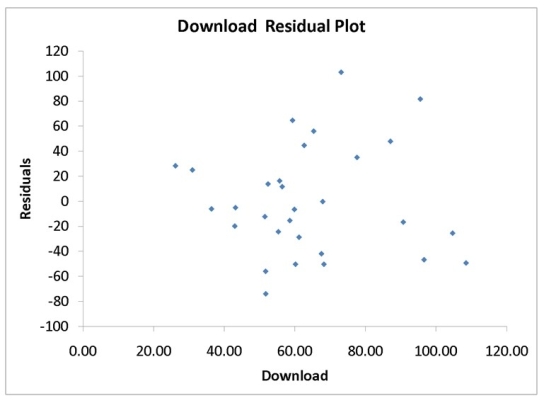
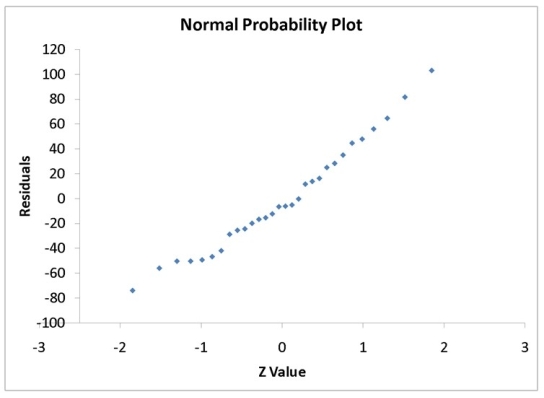
-Referring to Instruction 12.19,which of the following assumptions appears to have been violated?

• A) Independence of errors
• B) Normality of error
• C) Homoscedasticity
• D) None of the above

---

Definitions:

Complementary Relationship

A situation where two goods or services are used together, and the increase in consumption of one leads to an increase in consumption of the other.

Illegal Fruit Pickers

Individuals who are employed in agricultural harvesting without legal authorization or documentation.

Domestic-born Truck Drivers

Truck drivers who are born within the country they are working, as opposed to those who have immigrated.

Backflows

The reverse movement of water, mixtures, or substances into the distribution pipes of the potable supply of water from any source or sources.