Solved

Instruction 12 $\text { Regression statistics }$ $\text { ANOVA }$

question 38

Essay

Instruction 12.25
A computer software developer would like to use the number of downloads (in thousands) for the trial version of his new shareware to predict the amount of revenue (in thousands of dollars) he can make on the full version of the new shareware. Following is the output from a simple linear regression along with the residual plot and normal probability plot obtained from a data set of 30 different sharewares that he has developed:
$\text { Regression statistics }$
$\begin{array}{|l|l|}\hline \text { MultipleR } & 0.8691 \\\hline \text { R Square } & 0.7554 \\\hline \begin{array}{l}\text { Adjusted R } \\\text { Square }\end{array} & 0.7467 \\\hline \text { Standard Error } & 44.4765 \\\hline \text { Observations } & 30.0000\\\hline \end{array}$

$\text { ANOVA }$
$\begin{array}{|l|l|l|l|l|l|}\hline & d f & S S & M S & F & \begin{array}{l}\text { Significance } \\F\end{array} \\\hline \text { Regression } & 1 & 171062.9193 & 171062.9193 & 86.4759 & 0.0000 \\\hline \text { Residual } & 28 & 55388.4309 & 1978.1582 & & \\\hline \text { Total } & 29 & 226451.3503 & & & \\\hline\end{array}$

$\begin{array}{|l|l|l|l|l|l|l|}\hline & \text { Coefficients } & \begin{array}{l}\text { Standard } \\\text { Error }\end{array} & \text { t Stat } & p \text {-value } & \text { Lower 95\% } & \text { Upper 95\% } \\\hline \text { Intercept } & -95.0614 & 26.9183 & -3.5315 & 0.0015 & -150.2009 & -39.9218 \\\hline \text { Download } & 3.7297 & 0.4011 & 9.2992 & 0.0000 & 2.9082 & 4.5513 \\\hline\end{array}$

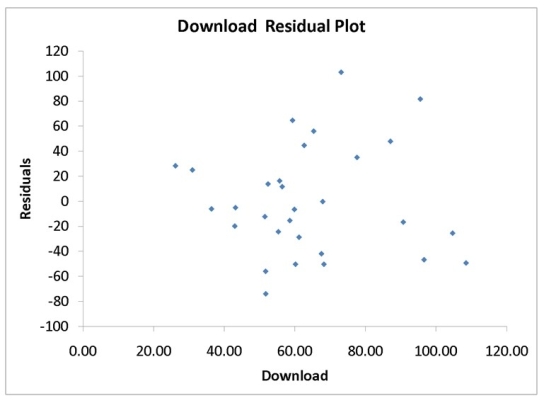
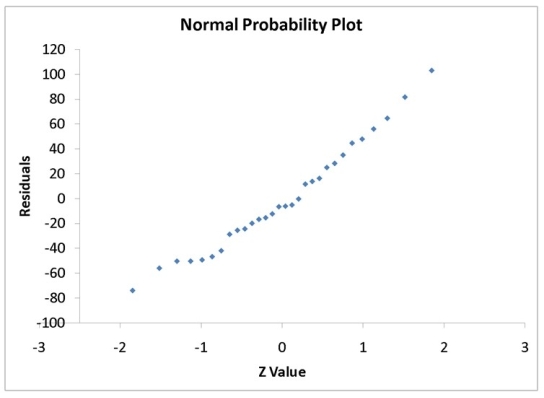
$Instruction 12.25 A computer software developer would like to use the number of downloads (in thousands) for the trial version of his new shareware to predict the amount of revenue (in thousands of dollars) he can make on the full version of the new shareware. Following is the output from a simple linear regression along with the residual plot and normal probability plot obtained from a data set of 30 different sharewares that he has developed: \text { Regression statistics } \begin{array}{|l|l|} \hline \text { MultipleR } & 0.8691 \\ \hline \text { R Square } & 0.7554 \\ \hline \begin{array}{l} \text { Adjusted R } \\ \text { Square } \end{array} & 0.7467 \\ \hline \text { Standard Error } & 44.4765 \\ \hline \text { Observations } & 30.0000\\ \hline \end{array} \text { ANOVA } \begin{array}{|l|l|l|l|l|l|} \hline & d f & S S & M S & F & \begin{array}{l} \text { Significance } \\ F \end{array} \\ \hline \text { Regression } & 1 & 171062.9193 & 171062.9193 & 86.4759 & 0.0000 \\ \hline \text { Residual } & 28 & 55388.4309 & 1978.1582 & & \\ \hline \text { Total } & 29 & 226451.3503 & & & \\ \hline \end{array} \begin{array}{|l|l|l|l|l|l|l|} \hline & \text { Coefficients } & \begin{array}{l} \text { Standard } \\ \text { Error } \end{array} & \text { t Stat } & p \text {-value } & \text { Lower 95\% } & \text { Upper 95\% } \\ \hline \text { Intercept } & -95.0614 & 26.9183 & -3.5315 & 0.0015 & -150.2009 & -39.9218 \\ \hline \text { Download } & 3.7297 & 0.4011 & 9.2992 & 0.0000 & 2.9082 & 4.5513 \\ \hline \end{array} -Referring to Instruction 12.25,the Durbin-Watson statistic is inappropriate for this data set.$ $Instruction 12.25 A computer software developer would like to use the number of downloads (in thousands) for the trial version of his new shareware to predict the amount of revenue (in thousands of dollars) he can make on the full version of the new shareware. Following is the output from a simple linear regression along with the residual plot and normal probability plot obtained from a data set of 30 different sharewares that he has developed: \text { Regression statistics } \begin{array}{|l|l|} \hline \text { MultipleR } & 0.8691 \\ \hline \text { R Square } & 0.7554 \\ \hline \begin{array}{l} \text { Adjusted R } \\ \text { Square } \end{array} & 0.7467 \\ \hline \text { Standard Error } & 44.4765 \\ \hline \text { Observations } & 30.0000\\ \hline \end{array} \text { ANOVA } \begin{array}{|l|l|l|l|l|l|} \hline & d f & S S & M S & F & \begin{array}{l} \text { Significance } \\ F \end{array} \\ \hline \text { Regression } & 1 & 171062.9193 & 171062.9193 & 86.4759 & 0.0000 \\ \hline \text { Residual } & 28 & 55388.4309 & 1978.1582 & & \\ \hline \text { Total } & 29 & 226451.3503 & & & \\ \hline \end{array} \begin{array}{|l|l|l|l|l|l|l|} \hline & \text { Coefficients } & \begin{array}{l} \text { Standard } \\ \text { Error } \end{array} & \text { t Stat } & p \text {-value } & \text { Lower 95\% } & \text { Upper 95\% } \\ \hline \text { Intercept } & -95.0614 & 26.9183 & -3.5315 & 0.0015 & -150.2009 & -39.9218 \\ \hline \text { Download } & 3.7297 & 0.4011 & 9.2992 & 0.0000 & 2.9082 & 4.5513 \\ \hline \end{array} -Referring to Instruction 12.25,the Durbin-Watson statistic is inappropriate for this data set.$
-Referring to Instruction 12.25,the Durbin-Watson statistic is inappropriate for this data set.

Definitions:

Bankruptcy

A legal proceeding involving a person or business that is unable to repay their outstanding debts, allowing for the liquidation or reorganization of assets to pay off creditors.

Voidable Preference

A legal term referring to a payment or asset transfer made by a debtor that can be invalidated by a bankruptcy trustee under certain conditions.

Insider

An individual with access to confidential information about a company, often an employee or executive, which can affect financial decisions or market performance.

Liquidation

The process of converting a company's assets into cash to pay off creditors before ceasing operations or redistributing assets to shareholders.

Instruction 12 Regression statistics \text { Regression statistics } Regression statistics ANOVA \text { ANOVA } ANOVA

Definitions:

Instruction 12 $\text { Regression statistics }$ $\text { ANOVA }$