Solved

Instruction 12 $\text { Regression statistics }$ $\text { ANOVA }$

question 149

Short Answer

Instruction 12.17
A computer software developer would like to use the number of downloads (in thousands) for the trial version of his new shareware to predict the amount of revenue (in thousands of dollars) he can make on the full version of the new shareware. Following is the output from a simple linear regression along with the residual plot and normal probability plot obtained from a data set of 30 different sharewares that he has developed:
$\text { Regression statistics }$
$\begin{array}{|l|l|}\hline \text { MultipleR } & 0.8691 \\\hline \text { R Square } & 0.7554 \\\hline \begin{array}{l}\text { Adjusted R } \\\text { Square }\end{array} & 0.7467 \\\hline \text { Standard Error } & 44.4765 \\\hline \text { Observations } & 30.0000\\\hline \end{array}$

$\text { ANOVA }$
$\begin{array}{|l|l|l|l|l|l|}\hline & d f & S S & M S & F & \begin{array}{l}\text { Significance } \\F\end{array} \\\hline \text { Regression } & 1 & 171062.9193 & 171062.9193 & 86.4759 & 0.0000 \\\hline \text { Residual } & 28 & 55388.4309 & 1978.1582 & & \\\hline \text { Total } & 29 & 226451.3503 & & & \\\hline\end{array}$

$\begin{array}{|l|l|l|l|l|l|l|}\hline & \text { Coefficients } & \begin{array}{l}\text { Standard } \\\text { Error }\end{array} & \text { t Stat } & p \text {-value } & \text { Lower 95\% } & \text { Upper 95\% } \\\hline \text { Intercept } & -95.0614 & 26.9183 & -3.5315 & 0.0015 & -150.2009 & -39.9218 \\\hline \text { Download } & 3.7297 & 0.4011 & 9.2992 & 0.0000 & 2.9082 & 4.5513 \\\hline\end{array}$
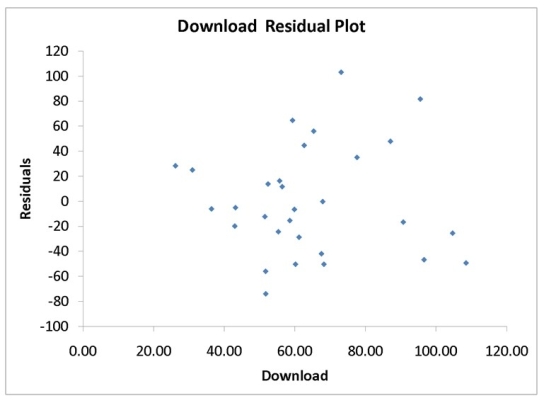
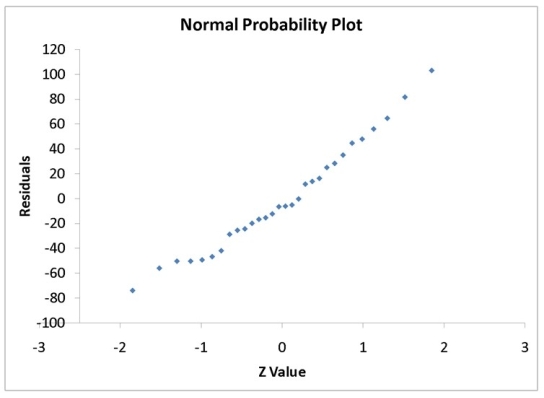
$Instruction 12.17 A computer software developer would like to use the number of downloads (in thousands) for the trial version of his new shareware to predict the amount of revenue (in thousands of dollars) he can make on the full version of the new shareware. Following is the output from a simple linear regression along with the residual plot and normal probability plot obtained from a data set of 30 different sharewares that he has developed: \text { Regression statistics } \begin{array}{|l|l|} \hline \text { MultipleR } & 0.8691 \\ \hline \text { R Square } & 0.7554 \\ \hline \begin{array}{l} \text { Adjusted R } \\ \text { Square } \end{array} & 0.7467 \\ \hline \text { Standard Error } & 44.4765 \\ \hline \text { Observations } & 30.0000\\ \hline \end{array} \text { ANOVA } \begin{array}{|l|l|l|l|l|l|} \hline & d f & S S & M S & F & \begin{array}{l} \text { Significance } \\ F \end{array} \\ \hline \text { Regression } & 1 & 171062.9193 & 171062.9193 & 86.4759 & 0.0000 \\ \hline \text { Residual } & 28 & 55388.4309 & 1978.1582 & & \\ \hline \text { Total } & 29 & 226451.3503 & & & \\ \hline \end{array} \begin{array}{|l|l|l|l|l|l|l|} \hline & \text { Coefficients } & \begin{array}{l} \text { Standard } \\ \text { Error } \end{array} & \text { t Stat } & p \text {-value } & \text { Lower 95\% } & \text { Upper 95\% } \\ \hline \text { Intercept } & -95.0614 & 26.9183 & -3.5315 & 0.0015 & -150.2009 & -39.9218 \\ \hline \text { Download } & 3.7297 & 0.4011 & 9.2992 & 0.0000 & 2.9082 & 4.5513 \\ \hline \end{array} -Referring to Instruction 12.17,what is the standard error of estimate?$ $Instruction 12.17 A computer software developer would like to use the number of downloads (in thousands) for the trial version of his new shareware to predict the amount of revenue (in thousands of dollars) he can make on the full version of the new shareware. Following is the output from a simple linear regression along with the residual plot and normal probability plot obtained from a data set of 30 different sharewares that he has developed: \text { Regression statistics } \begin{array}{|l|l|} \hline \text { MultipleR } & 0.8691 \\ \hline \text { R Square } & 0.7554 \\ \hline \begin{array}{l} \text { Adjusted R } \\ \text { Square } \end{array} & 0.7467 \\ \hline \text { Standard Error } & 44.4765 \\ \hline \text { Observations } & 30.0000\\ \hline \end{array} \text { ANOVA } \begin{array}{|l|l|l|l|l|l|} \hline & d f & S S & M S & F & \begin{array}{l} \text { Significance } \\ F \end{array} \\ \hline \text { Regression } & 1 & 171062.9193 & 171062.9193 & 86.4759 & 0.0000 \\ \hline \text { Residual } & 28 & 55388.4309 & 1978.1582 & & \\ \hline \text { Total } & 29 & 226451.3503 & & & \\ \hline \end{array} \begin{array}{|l|l|l|l|l|l|l|} \hline & \text { Coefficients } & \begin{array}{l} \text { Standard } \\ \text { Error } \end{array} & \text { t Stat } & p \text {-value } & \text { Lower 95\% } & \text { Upper 95\% } \\ \hline \text { Intercept } & -95.0614 & 26.9183 & -3.5315 & 0.0015 & -150.2009 & -39.9218 \\ \hline \text { Download } & 3.7297 & 0.4011 & 9.2992 & 0.0000 & 2.9082 & 4.5513 \\ \hline \end{array} -Referring to Instruction 12.17,what is the standard error of estimate?$
-Referring to Instruction 12.17,what is the standard error of estimate?

Definitions:

Pollution Abatement

Measures and strategies implemented to reduce, manage, and control environmental pollution.

Marginal Social Cost

The total cost to society of producing an additional unit of a good or service, including private costs and any externalities.

Emissions Control

Regulations and technologies designed to reduce pollution and greenhouse gas emissions from various sources.

Marginal Social Costs

The additional cost incurred by society as a whole from the production of one more unit of a good or service.

Instruction 12 Regression statistics \text { Regression statistics } Regression statistics ANOVA \text { ANOVA } ANOVA

Definitions:

Instruction 12 $\text { Regression statistics }$ $\text { ANOVA }$