Solved

Instruction 12 $\text { Regression statistics }$ $\text { ANOVA }$

question 2

Short Answer

Instruction 12.17
A computer software developer would like to use the number of downloads (in thousands) for the trial version of his new shareware to predict the amount of revenue (in thousands of dollars) he can make on the full version of the new shareware. Following is the output from a simple linear regression along with the residual plot and normal probability plot obtained from a data set of 30 different sharewares that he has developed:
$\text { Regression statistics }$
$\begin{array}{|l|l|}\hline \text { MultipleR } & 0.8691 \\\hline \text { R Square } & 0.7554 \\\hline \begin{array}{l}\text { Adjusted R } \\\text { Square }\end{array} & 0.7467 \\\hline \text { Standard Error } & 44.4765 \\\hline \text { Observations } & 30.0000\\\hline \end{array}$

$\text { ANOVA }$
$\begin{array}{|l|l|l|l|l|l|}\hline & d f & S S & M S & F & \begin{array}{l}\text { Significance } \\F\end{array} \\\hline \text { Regression } & 1 & 171062.9193 & 171062.9193 & 86.4759 & 0.0000 \\\hline \text { Residual } & 28 & 55388.4309 & 1978.1582 & & \\\hline \text { Total } & 29 & 226451.3503 & & & \\\hline\end{array}$

$\begin{array}{|l|l|l|l|l|l|l|}\hline & \text { Coefficients } & \begin{array}{l}\text { Standard } \\\text { Error }\end{array} & \text { t Stat } & p \text {-value } & \text { Lower 95\% } & \text { Upper 95\% } \\\hline \text { Intercept } & -95.0614 & 26.9183 & -3.5315 & 0.0015 & -150.2009 & -39.9218 \\\hline \text { Download } & 3.7297 & 0.4011 & 9.2992 & 0.0000 & 2.9082 & 4.5513 \\\hline\end{array}$
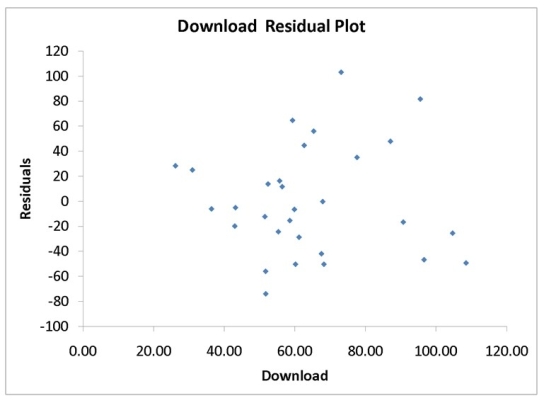
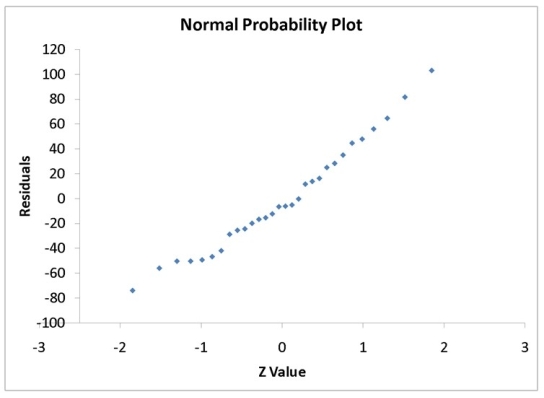
$Instruction 12.17 A computer software developer would like to use the number of downloads (in thousands) for the trial version of his new shareware to predict the amount of revenue (in thousands of dollars) he can make on the full version of the new shareware. Following is the output from a simple linear regression along with the residual plot and normal probability plot obtained from a data set of 30 different sharewares that he has developed: \text { Regression statistics } \begin{array}{|l|l|} \hline \text { MultipleR } & 0.8691 \\ \hline \text { R Square } & 0.7554 \\ \hline \begin{array}{l} \text { Adjusted R } \\ \text { Square } \end{array} & 0.7467 \\ \hline \text { Standard Error } & 44.4765 \\ \hline \text { Observations } & 30.0000\\ \hline \end{array} \text { ANOVA } \begin{array}{|l|l|l|l|l|l|} \hline & d f & S S & M S & F & \begin{array}{l} \text { Significance } \\ F \end{array} \\ \hline \text { Regression } & 1 & 171062.9193 & 171062.9193 & 86.4759 & 0.0000 \\ \hline \text { Residual } & 28 & 55388.4309 & 1978.1582 & & \\ \hline \text { Total } & 29 & 226451.3503 & & & \\ \hline \end{array} \begin{array}{|l|l|l|l|l|l|l|} \hline & \text { Coefficients } & \begin{array}{l} \text { Standard } \\ \text { Error } \end{array} & \text { t Stat } & p \text {-value } & \text { Lower 95\% } & \text { Upper 95\% } \\ \hline \text { Intercept } & -95.0614 & 26.9183 & -3.5315 & 0.0015 & -150.2009 & -39.9218 \\ \hline \text { Download } & 3.7297 & 0.4011 & 9.2992 & 0.0000 & 2.9082 & 4.5513 \\ \hline \end{array} -Referring to Instruction 12.17,what is the standard deviation around the regression line?$ $Instruction 12.17 A computer software developer would like to use the number of downloads (in thousands) for the trial version of his new shareware to predict the amount of revenue (in thousands of dollars) he can make on the full version of the new shareware. Following is the output from a simple linear regression along with the residual plot and normal probability plot obtained from a data set of 30 different sharewares that he has developed: \text { Regression statistics } \begin{array}{|l|l|} \hline \text { MultipleR } & 0.8691 \\ \hline \text { R Square } & 0.7554 \\ \hline \begin{array}{l} \text { Adjusted R } \\ \text { Square } \end{array} & 0.7467 \\ \hline \text { Standard Error } & 44.4765 \\ \hline \text { Observations } & 30.0000\\ \hline \end{array} \text { ANOVA } \begin{array}{|l|l|l|l|l|l|} \hline & d f & S S & M S & F & \begin{array}{l} \text { Significance } \\ F \end{array} \\ \hline \text { Regression } & 1 & 171062.9193 & 171062.9193 & 86.4759 & 0.0000 \\ \hline \text { Residual } & 28 & 55388.4309 & 1978.1582 & & \\ \hline \text { Total } & 29 & 226451.3503 & & & \\ \hline \end{array} \begin{array}{|l|l|l|l|l|l|l|} \hline & \text { Coefficients } & \begin{array}{l} \text { Standard } \\ \text { Error } \end{array} & \text { t Stat } & p \text {-value } & \text { Lower 95\% } & \text { Upper 95\% } \\ \hline \text { Intercept } & -95.0614 & 26.9183 & -3.5315 & 0.0015 & -150.2009 & -39.9218 \\ \hline \text { Download } & 3.7297 & 0.4011 & 9.2992 & 0.0000 & 2.9082 & 4.5513 \\ \hline \end{array} -Referring to Instruction 12.17,what is the standard deviation around the regression line?$
-Referring to Instruction 12.17,what is the standard deviation around the regression line?

Definitions:

Microsoft Publisher

A desktop publishing application by Microsoft, designed for creating professional-quality documents such as brochures and newsletters.

Cut and Pasting

A method of moving or copying text, files, or data from one location to another within digital documents or between software applications.

Word 2010

A version of Microsoft Word, part of the Microsoft Office 2010 suite, that includes enhanced features for document creation, editing, and formatting.

Save Options

Features in software applications that allow users to save their progress, work, or data in various formats or locations.

Instruction 12 Regression statistics \text { Regression statistics } Regression statistics ANOVA \text { ANOVA } ANOVA

Definitions:

Instruction 12 $\text { Regression statistics }$ $\text { ANOVA }$