The Volume of Helium in a Spherical Balloon (In Cubic

question 147

Short Answer

The volume of helium in a spherical balloon (in cubic centimeters)as a function of the radius,r,in centimeters,is given by V(r)=
π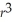
.Graph V(r)in the standard viewing rectangle,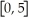
×
and use TRACE to estimate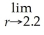
V(r).

---

Definitions:

Level

The specified significance level in a statistical test, often denoted as alpha, which is the probability of rejecting the null hypothesis when it is actually true.

One-Way ANOVA

A statistical test that analyzes the differences among group means in a sample, used when comparing three or more groups for a single independent variable.

Population Means

The average values of multiple populations, each calculated by adding all members of each population and then dividing by the number of members in that population.

Goblet Cells

Specialized, mucus-producing cells found in the respiratory and intestinal tracts, which help to protect and lubricate the surfaces.