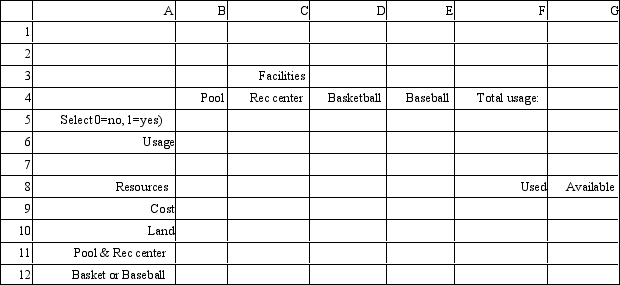
A Small Town Wants to Build Some New Recreational Facilities

question 34

Essay

A small town wants to build some new recreational facilities.The proposed facilities include a swimming pool,recreation center,basketball court and baseball field.The town council wants to provide the facilities which will be used by the most people,but faces budget and land limitations.The town has $400,000 and 14 acres of land.The pool requires locker facilities which would be in the recreation center,so if the swimming pool is built the recreation center must also be built.Also the council has only enough flat land to build the basketball court or the baseball field.The daily usage and cost of the facilities in $1,000)are shown below.
$$\begin{array} { r r r r r } \text { Variable } & \text { Facilty } & \text { Usage } & \text { Cost \$1,000) } & \text { Land } \\\hline \mathbf { x } _ { 1 } & \text { Swimming pool } & 400 & 100 & 2 \\\mathbf { x } _ { 2 } & \text { Recreation center } & 500 & 200 & 3 \\\mathbf { x } _ { 3 } & \text { Basketball court } & 300 & 150 & 4 \\\mathbf { x } _ { 4 } & \text { Baseball field } & 200 & 100 & 5\end{array}$$
Based on this ILP formulation of the problem and the indicated optimal solution what values should go in cells
B5:G12 of the following Excel spreadsheet?
$$\begin{array} { l l l } \text { MAX: } & 400 \mathbf { x } _ { 1 } + 500 \mathbf { x } _ { 2 } + 300 \mathbf { x } _ { 3 } + 200 \mathbf { x } _ { 4 } & \\\text { Subject ta: } & 100 \mathbf { x } _ { 1 } + 200 \mathbf { x } _ { 2 } + 150 \mathbf { x } _ { 3 } + 100 \mathbf { x } _ { 4 } \leq 400 & \text { budget } \\& 2 \mathbf { x } _ { 1 } + 3 \mathbf { x } _ { 2 } + 4 \mathbf { x } _ { 3 } + 5 \mathbf { x } _ { 4 } \leq 14 & \text { land } \\& \mathbf { x } _ { 1 } - \mathbf { x } _ { 2 } \leq 0 & \text { pool and recreation center } \\& \mathbf { x } _ { 3 } + \mathbf { x } _ { 4 } \leq 1 & \text { basketball and baseball } \\& \mathbf { x } _ { 1 } = 0.1 &\end{array}$$
Solution: $\left. \mathbf { x } _ { 1 } , \mathbf { x } _ { 2 } , \mathbf { x } _ { 3 } , \mathbf { X } _ { 4 } \right) = 1,1,0,1$ )

---

Definitions:

Opportunity Cost

Forgoing possible benefits from other options by selecting a particular one.

Straight Lines

Lines in a geometric space that have the same direction throughout and no curvature.

Foreign Trade

The exchange of goods and services between countries.

Economic Output

The total value of all goods and services produced by an economy over a specified period of time.