Instruction 12-11
a Computer Software Developer Would Like to Use

question 42

Short Answer

Instruction 12-11
A computer software developer would like to use the number of downloads (in thousands)for the trial version of his new shareware to predict the amount of revenue (in thousands of dollars)he can make on the full version of the new shareware.Following is the output from a simple linear regression along with the residual plot and normal probability plot obtained from a data set of 30 different sharewares that he has developed:
$\begin{array}{l|r}\hline {\text { Regression Statistics }} \\\hline \text { Multiple R } & 0.8691 \\\hline \text { R Square } & 0.7554 \\\hline \text { Adjusted R Square } & 0.7467 \\\hline \text { Standard Error } & 44.4765 \\\hline \text { Observations } & 30.0000 \\\hline\end{array}$
ANOVA
$\begin{array}{|l|r|r|r|r|r|}\hline &df&SS&MS&F&significance F\\\hline \text { Regression } & 1 & 171062.9193 & 171062.9193 & 86.4759 & 0.0000 \\\hline \text { Residual } & 28 & 55388.4309 & 1978.1582 & & \\\hline \text { Total } & 29 & 226451.3503 & & & \\\hline\end{array}$

$\begin{array}{lrrrrrrr}\hline & \text { Coefficients } & \text { Standard Error } & t \text { Stat } & \text { P-value } & \text { Lower 95\% } & \text { Upper 95\% } \\\hline \text { Intercept } & -95.0614 & 26.9183 & -3.5315 & 0.0015 & -150.2009 & -39.9218 \\\text { Download } & 3.7297 & 0.4011 & 9.2992 & 0.0000 & 2.9082 & 4.5513 \\\hline\end{array}$
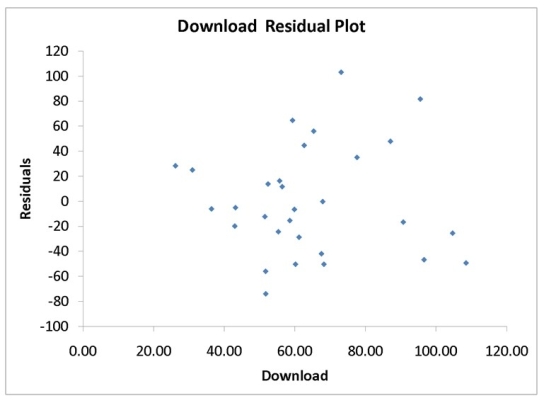
-Referring to Instruction 12-11,predict the revenue when the number of downloads is 30 thousand.

Understand the differences and purposes of various business reports (informational, analytical, proposals).

Distinguish between internal and external reports and their implications.

Recognize the components and significance of a business plan.

Understand the roles of direct and indirect approaches in business communication.

---

Definitions:

Hypothalamus

A region of the brain that controls an enormous number of bodily functions including hunger, thirst, sleep, and emotional activity.

Consciousness

The condition in which a person is conscious and can reflect on their own being, feelings, ideas, environment, and life events.

Suprachiasmatic Nucleus

A region in the brain's hypothalamus responsible for controlling circadian rhythms, including the sleep-wake cycle.

Circadian Cycle

The biological clock of living beings, regulating physiological and metabolic processes in a roughly 24-hour cycle.