Instruction 12-11
a Computer Software Developer Would Like to Use

question 171

True/False

Instruction 12-11
A computer software developer would like to use the number of downloads (in thousands)for the trial version of his new shareware to predict the amount of revenue (in thousands of dollars)he can make on the full version of the new shareware.Following is the output from a simple linear regression along with the residual plot and normal probability plot obtained from a data set of 30 different sharewares that he has developed:
$\begin{array}{lr}\hline {\text { Regression Statistics }} \\\hline \text { Multiple R } & 0.8691 \\\hline \text { R Square } & 0.7554 \\\hline \text { Adjusted R Square } & 0.7467 \\\hline \text { Standard Error } & 44.4765 \\\hline \text { Observations } & 30.0000 \\\hline\end{array}$
ANOVA
$\begin{array}{|l|r|r|r|r|r|}& d f & \text { SS } & {M S} & F & \text { Significance } F \\\hline \text { Regression } & 1 & 171062.9193 & 171062.9193 & 86.4759 & 0.0000 \\\hline \text { Residuall } & 28 & 55388.4309 & 1978.1582 & \\\hline \text { Total } & 29 & 226451.3503 & &\end{array}$

$\begin{array}{lrrrrrrr}\hline & \text { Coefficients } & \text { Standard Eror } &{t \text { Stat }} & \text { P-value } & \text { Lower 95\% } & \text { Upper 95\% } \\\hline \text { Intercept } & -95.0614 & 26.9183 & -3.5315 & 0.0015 & -150.2009 & -39.9218 \\\hline \text { Download } & 3.7297 & 0.4011 & 9.2992 & 0.0000 & 2.9082 & 4.5513\end{array}$
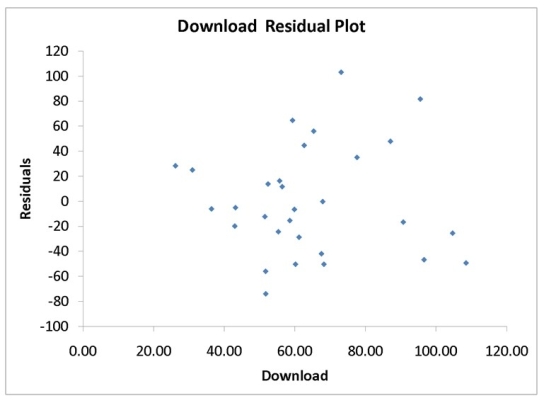
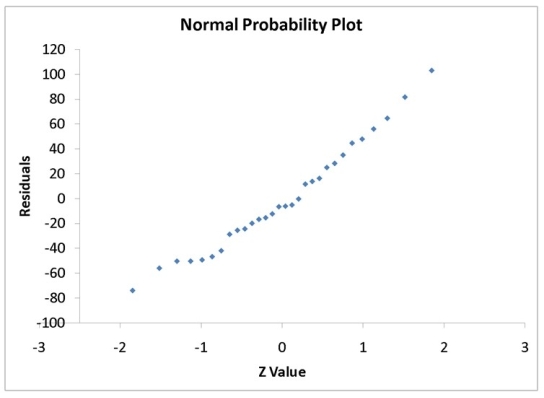
-Referring to Instruction 12-11,the null hypothesis for testing whether there is a linear relationship between revenue and number of dowloads is "There is no linear relationship between revenue and number of downloads".

---

Definitions:

Early Majority

A group in the diffusion of innovation model, representing consumers who adopt new products or technologies just before the average person and after the innovators and early adopters.

Microfibre Shirts

Clothing made from microfiber, a fine synthetic fiber known for its softness, durability, and moisture-wicking properties.

Advergaming

is a marketing strategy where video games are used to promote a brand, product, or message.

Advertising Pop-Ups

Advertising pop-ups are digital advertisements that appear over or on top of website content, often interrupting the user's viewing experience to capture attention.