Instruction 12-11
a Computer Software Developer Would Like to Use

question 180

True/False

Instruction 12-11
A computer software developer would like to use the number of downloads (in thousands)for the trial version of his new shareware to predict the amount of revenue (in thousands of dollars)he can make on the full version of the new shareware.Following is the output from a simple linear regression along with the residual plot and normal probability plot obtained from a data set of 30 different sharewares that he has developed:
$\begin{array}{lr}\hline {\text { Regression Statistics }} \\\hline \text { Multiple R } & 0.8691 \\\hline \text { R Square } & 0.7554 \\\hline \text { Adjusted R Square } & 0.7467 \\\hline \text { Standard Error } & 44.4765 \\\hline \text { Observations } & 30.0000 \\\hline\end{array}$
ANOVA
$\begin{array}{|l|r|r|r|r|r|}& d f & \text { SS } & {M S} & F & \text { Significance } F \\\hline \text { Regression } & 1 & 171062.9193 & 171062.9193 & 86.4759 & 0.0000 \\\hline \text { Residuall } & 28 & 55388.4309 & 1978.1582 & \\\hline \text { Total } & 29 & 226451.3503 & &\end{array}$

$\begin{array}{lrrrrrrr}\hline & \text { Coefficients } & \text { Standard Eror } &{t \text { Stat }} & \text { P-value } & \text { Lower 95\% } & \text { Upper 95\% } \\\hline \text { Intercept } & -95.0614 & 26.9183 & -3.5315 & 0.0015 & -150.2009 & -39.9218 \\\hline \text { Download } & 3.7297 & 0.4011 & 9.2992 & 0.0000 & 2.9082 & 4.5513\end{array}$
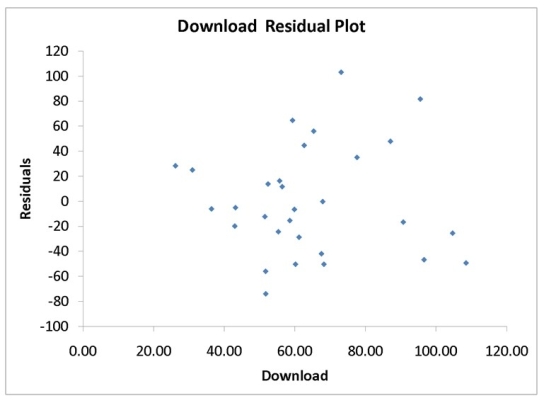
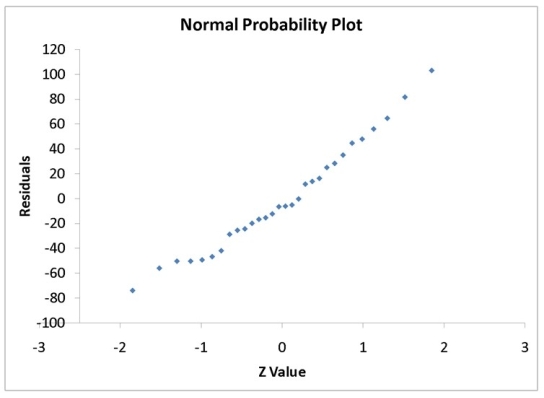
-Referring to Instruction 12-11,there is sufficient evidence that revenue and number of downloads are linearly related at a 5% level of significance.

Familiarize with the concept of short selling and futures positions in financial markets.

Grasp the cash flow characteristics and rights of preferred stockholders versus common stockholders.

Identify the components of major international market indexes.

Understand securitization and its examples in the financial markets.

---

Definitions:

December

The last month of the year according to the Gregorian calendar, which is the twelfth.

Cash Disbursements

Payments made by a business in cash or cash equivalents, including expenses, debt payments, and distributions to owners.

Net Income

The total profit or loss of a company after all expenses, including taxes and interest, have been deducted from total revenue.

December

The twelfth and final month of the year in the Gregorian calendar, frequently associated with end-of-year summaries and holiday seasons.