True/False
The probability density function of a continuous random variable X is , . Is this the graph of f(x) with the shaded area corresponding to ? 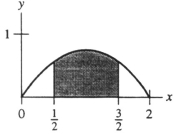
Definitions:
Related Questions
Q17: 2 ln x + 3 ln
Q24: Find the equation of the tangent
Q52: <span class="ql-formula" data-value="\sqrt { \ln 3 x
Q53: An automobile's brakes are applied at time
Q67: A car dealer records the number of
Q80: During the month of February, a
Q109: <span class="ql-formula" data-value="e ^ { 2 }
Q115: Determine the average value of f(x)
Q123: Which of the following series converge?<br>A)
Q176: <span class="ql-formula" data-value="\lim _ { x \rightarrow