Assume That Each Nurse Works 8 Consecutive Hours at the Beaver

question 70

Multiple Choice

Assume that each nurse works 8 consecutive hours at the Beaver Medical Center. The center has the following staffing requirements for each 4-hour work period. ​
Work Period
Nurses Needed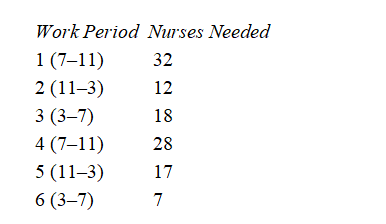

If represents the number of nurses starting in period 1, the number starting in period 2, and so on, write and solve the linear programming problem that will minimize the total number of nurses needed. (Note that the nurses who begin work in period 6 work periods 6 and 1 for their 8-hour shift.)
​

• A) The minimum 67 is nurses with 27 nurses starting in period 1, 5 nurses starting in period 2, 18 nurses starting in period 3, 10 nurses starting in period 4, and 7 nurses starting in period 5 (no nurses need to start in period 6).
• B) The minimum is 67 nurses with 27 nurses starting in period 1, 18 nurses starting in period 3, and 10 nurses starting in period 4, 7 nurses starting in period 5, and 5 nurses starting in period 6 (no nurses need to start in period 2).
• C) The minimum is 67 nurses with 32 nurses starting in period 1, 18 nurses starting in period 3, and 10 nurses starting in period 4, and 7 nurses starting in period 5 (no nurses need to start in periods 2 and 6).
• D) The minimum is 72 nurses with 32 nurses starting in period 1, 18 nurses starting in period 3, and 10 nurses starting in period 4, and 12 nurses starting in period 5 (no nurses need to start in periods 2 and 6).
• E) The minimum is 62 nurses with 32 nurses starting in period 1, 13 nurses starting in period 3, and 10 nurses starting in period 4, and 7 nurses starting in period 5 (no nurses need to start in periods 2 and 6).

---

Definitions:

Optimal Risky Portfolio

The optimal risky portfolio is the combination of risky assets that provides the highest expected return for a given level of risk or the lowest risk for a given level of expected return.

Risk Free Rate

The theoretical rate of return of an investment with zero risk, often represented by the yield on government securities like U.S. Treasury bonds.

Standard Deviation

A statistical measure that quantifies the variation or dispersion of a set of data points.

Optimal Risky Portfolio

A portfolio that offers the highest expected return for a given level of risk or the lowest risk for a given level of expected return, according to modern portfolio theory.