Bernoulli's equation for nonviscous flow can be stated as
P1 + gy1 + 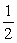 v12 = P2 + gy2 +
v12 = P2 + gy2 + 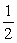 v22
v22
A fluid is flowing through a horizontal tube that changes in cross-sectional area from
A1= 0.75 cm2 to A2 = 0.030 cm2. 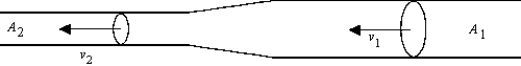 When v1 = 3.5 cm/s, = 1.4 g/cm3, and viscosity is neglected, the difference between pressure P2 at A2 and P1 at A1 is
When v1 = 3.5 cm/s, = 1.4 g/cm3, and viscosity is neglected, the difference between pressure P2 at A2 and P1 at A1 is
Definitions:
Legal Entity
Any organization that possesses separate legal personality from its members, such as corporations, partnerships, and sole proprietorships.
Personal Liability
The legal responsibility of an individual to bear the cost of damages or debt incurred through their actions or ownership.
Sole Proprietorship
A business owned and operated by a single individual, with no legal distinction between the owner and the business.
Business for Profit
Organizations that operate with the primary goal of earning financial returns for their owners or shareholders.
Q2: Which of the following statements concerning the
Q6: The graph shows the average power delivered
Q8: <img src="https://d2lvgg3v3hfg70.cloudfront.net/TB6080/.jpg" alt=" Two loudspeakers S<sub>1</sub>
Q11: The Empire's space station is a
Q36: A piano wire has a tension
Q40: Two objects with masses M<sub>1</sub> =
Q44: <img src="https://d2lvgg3v3hfg70.cloudfront.net/TB6080/.jpg" alt=" Three solid, homogeneous
Q46: Momentum is conserved in which of the
Q48: A body is in mechanical equilibrium when<br>A)
Q125: If f<sub>o</sub> is the fundamental frequency, the