A Right Circular Cone Has Height Equal to 4 Times

question 41

Essay

A right circular cone has height equal to 4 times its radius. The radius of the cone is increasing at a constant rate. When the area of the base is increasing at a rate of 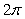square meters per second, the volume is increasing at a rate of 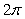cubic meters per second. If, at a later time, the area of the base is increasing at a rate of 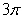square meters per second, how fast is the volume increasing? How fast is the height increasing?

Recognize the importance of matching individual interests and skills to job roles.

Describe the role and importance of performance reviews in organizational settings.

Differentiate among the roles of various types of psychologists within an organizational context.

Identify the key responsibilities and concerns of human factors psychology versus organizational psychology.