Solved

When a Spring Is Stretched and Then Released, It Oscillates

question 14

Multiple Choice

When a spring is stretched and then released, it oscillates according to two laws of physics: Hooke's Law and Netwon's Second Law. These two laws combine to form the following differential equation in the case of free, undamped oscillation: 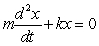where m is the mass of an object attached to the spring, x is the distance the spring is stretched beyond its standard length with the object attached (its equilibrium point) , t is time, and k is a constant associated with the strength of the spring. Consider a spring with 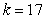from which is hung a 68-pound weight. The spring with the weight attached stretches to its equilibrium point. The spring is then pulled 3 feet farther than its equilibrium and released. Write a differential equation describing the acceleration of the spring with respect to time t measured in seconds. Use the fact that 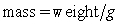, where g is the gravitational constant 32 feet per second per second.

Recognize different branded content approaches and their unique features.

Acknowledge the use and significance of location-based marketing and apps.

Understand the negative implications of internet censorship on brands.

Identify the role and techniques of social listening in managing company reputation online.

Definitions:

Ileum

The last portion of the small intestine. It is directly attached to the large intestine.

Small Intestine

A long, coiled part of the digestive system where most of the absorption of nutrients occurs.

Collagen

A protein that is a major component of connective tissues in the body, providing structural support and strength.

Absorption

The process by which substances are taken up by a tissue, such as the absorption of nutrients by the intestines.