Examlex

Solved

(Requires Appendix Material and Calculus)Equation (5

question 49

Essay

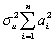(Requires Appendix material and Calculus)Equation (5.36)in your textbook derives the conditional variance for any old conditionally unbiased estimator (Requires Appendix material and Calculus)Equation (5.36)in your textbook derives the conditional variance for any old conditionally unbiased estimator 1 to be var( 1 X1,... ,Xn)= where the conditions for conditional unbiasedness are = 0 and = 1.As an alternative to the BLUE proof presented in your textbook,you recall from one of your calculus courses that you could minimize the variance subject to the two constraints,thereby making the variance as small as possible while the constraints are holding.Show that in doing so you get the OLS weights .(You may assume that X1,... ,Xn are nonrandom (fixed over repeated samples). 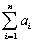) 1 to be var( (Requires Appendix material and Calculus)Equation (5.36)in your textbook derives the conditional variance for any old conditionally unbiased estimator 1 to be var( 1 X1,... ,Xn)= where the conditions for conditional unbiasedness are = 0 and = 1.As an alternative to the BLUE proof presented in your textbook,you recall from one of your calculus courses that you could minimize the variance subject to the two constraints,thereby making the variance as small as possible while the constraints are holding.Show that in doing so you get the OLS weights .(You may assume that X1,... ,Xn are nonrandom (fixed over repeated samples). 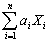) 1 (Requires Appendix material and Calculus)Equation (5.36)in your textbook derives the conditional variance for any old conditionally unbiased estimator 1 to be var( 1 X1,... ,Xn)= where the conditions for conditional unbiasedness are = 0 and = 1.As an alternative to the BLUE proof presented in your textbook,you recall from one of your calculus courses that you could minimize the variance subject to the two constraints,thereby making the variance as small as possible while the constraints are holding.Show that in doing so you get the OLS weights .(You may assume that X1,... ,Xn are nonrandom (fixed over repeated samples). ) X1,... 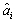,Xn)= (Requires Appendix material and Calculus)Equation (5.36)in your textbook derives the conditional variance for any old conditionally unbiased estimator 1 to be var( 1 X1,... ,Xn)= where the conditions for conditional unbiasedness are = 0 and = 1.As an alternative to the BLUE proof presented in your textbook,you recall from one of your calculus courses that you could minimize the variance subject to the two constraints,thereby making the variance as small as possible while the constraints are holding.Show that in doing so you get the OLS weights .(You may assume that X1,... ,Xn are nonrandom (fixed over repeated samples). ) where the conditions for conditional unbiasedness are (Requires Appendix material and Calculus)Equation (5.36)in your textbook derives the conditional variance for any old conditionally unbiased estimator 1 to be var( 1 X1,... ,Xn)= where the conditions for conditional unbiasedness are = 0 and = 1.As an alternative to the BLUE proof presented in your textbook,you recall from one of your calculus courses that you could minimize the variance subject to the two constraints,thereby making the variance as small as possible while the constraints are holding.Show that in doing so you get the OLS weights .(You may assume that X1,... ,Xn are nonrandom (fixed over repeated samples). ) = 0 and (Requires Appendix material and Calculus)Equation (5.36)in your textbook derives the conditional variance for any old conditionally unbiased estimator 1 to be var( 1 X1,... ,Xn)= where the conditions for conditional unbiasedness are = 0 and = 1.As an alternative to the BLUE proof presented in your textbook,you recall from one of your calculus courses that you could minimize the variance subject to the two constraints,thereby making the variance as small as possible while the constraints are holding.Show that in doing so you get the OLS weights .(You may assume that X1,... ,Xn are nonrandom (fixed over repeated samples). ) = 1.As an alternative to the BLUE proof presented in your textbook,you recall from one of your calculus courses that you could minimize the variance subject to the two constraints,thereby making the variance as small as possible while the constraints are holding.Show that in doing so you get the OLS weights (Requires Appendix material and Calculus)Equation (5.36)in your textbook derives the conditional variance for any old conditionally unbiased estimator 1 to be var( 1 X1,... ,Xn)= where the conditions for conditional unbiasedness are = 0 and = 1.As an alternative to the BLUE proof presented in your textbook,you recall from one of your calculus courses that you could minimize the variance subject to the two constraints,thereby making the variance as small as possible while the constraints are holding.Show that in doing so you get the OLS weights .(You may assume that X1,... ,Xn are nonrandom (fixed over repeated samples). ) .(You may assume that X1,... ,Xn are nonrandom (fixed over repeated samples). )

Describe conditions for allocative efficiency in markets.
Recognize the efficiency outcomes of competitive markets.
Understand public goods and their characteristics.
Identify the role of government in market economies.

Definitions:

Muscle Fibers

The individual cellular units of a muscle, consisting of long, slender cells capable of contraction to produce movement.

Muscle Length

The extent from the origin to the insertion of a muscle, determining its capacity to generate force and undergo contraction.

Adaptation

The process by which organisms adjust to changes in their environment over time, either through genetic changes or behaviorally, to improve survival and reproduction rates.

Continued Stimulus

A continued stimulus refers to a persistent or ongoing stimulus that can modify physiological or psychological responses over time.

Related Questions

Q3: The homoskedasticity-only estimator of the variance of

Q6: Derivatives permit investors to manage their risk

Q7: You can determine the lag lengths in

Q16: When the sample size n is large,the

Q19: Write the following three linear equations in

Q21: Consider the standard AR(1)Yt = β0 +

Q22: The Solow growth model suggests that countries

Q29: Consider the distributed lag model Yt =

Q33: In the simple linear regression model,the regression

Q58: Applying the analysis from the California test