A Researcher Is Investigating Variables That Might Be Associated with the Academic

question 90

Multiple Choice

A researcher is investigating variables that might be associated with the academic performance of high school students.She examined data from 1990 for each of the 50 states plus Washington,DC.The data included information on the following variables. As part of her investigation,she ran the multiple regression model SATM = ₀ + ₁($ per pupil) + ₂(% taking) + _i,
Where the deviations _i were assumed to be independent and Normally distributed with a mean of 0 and a standard deviation of .This model was fit to the data using the method of least squares.The following results were obtained from statistical software. 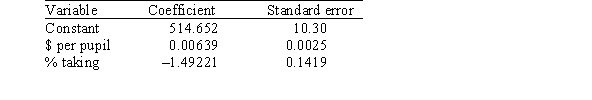Another researcher,using the same data,ran the simple linear regression model
SATM = ₀ + ₁($ per pupil) + _i.
The following results were obtained from statistical software. 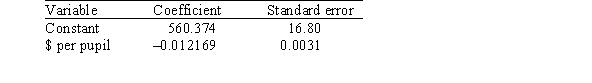The first researcher concluded that because the coefficient for the variable $ per pupil was positive in her results,spending additional money on students would have a positive effect on SATM scores.This researcher therefore recommended more money be spent on students.The second researcher concluded that because the coefficient for the variable $ per pupil was negative in his results,spending additional money on students would have a negative effect on SATM scores.This researcher therefore recommended less money be spent on students.Why are these two conclusions different even though the researchers used the same data?

• A)An error must have been made by one of the researchers.
• B)Both researchers failed to take into account that in their analyses,  ₁,the coefficient of the variable $ per pupil,was not statistically significant at even the 0.10 significance level.Hence,neither researcher could conclude that  ₁ was significantly different from zero.
• C)The researchers did not use the same set of explanatory variables in their models.
• D)There must have been an influential observation in the data,rendering the analyses inappropriate.

---

Definitions:

Dividends

Payments made to shareholders out of a corporation's earnings, typically distributed regularly (e.g., quarterly).

Four-figure Accuracy

A level of precision in numerical information or calculations that is rounded to or expressed in thousands.

Algebraic Expression

A statement of the mathematical operations to be carried out on a combination of numbers and variables.

Monomial

An expression containing only one term.