TABLE 14-8 A Financial Analyst Wanted to Examine the Relationship Between Salary

question 289

True/False

TABLE 14-8
A financial analyst wanted to examine the relationship between salary (in $1,000)and 2 variables: age
(X₁ = Age)and experience in the field (X₂ = Exper).He took a sample of 20 employees and obtained the following Microsoft Excel output: 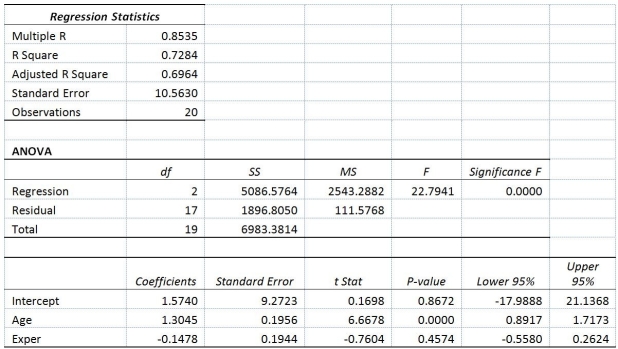Also,the sum of squares due to the regression for the model that includes only Age is 5022.0654 while the sum of squares due to the regression for the model that includes only Exper is 125.9848.
-True or False: Referring to Table 14-8,the analyst wants to use a t test to test for the significance of the coefficient of X₂.At a level of significance of 0.01,the department head would decide that β₂ ≠ 0.

---

Definitions:

Risk Premium

The additional return expected by an investor for taking on a higher level of risk compared to a risk-free investment.

Excess Returns

The return on an investment beyond the return expected from risk level, often used to assess performance.

Risk-free Asset

A theoretical financial instrument that promises a certain return with no risk of financial loss, often exemplified by government bonds.

Positively Skewed

Describes a distribution of data where the right tail (higher values) is longer than the left, indicating that a variable has more values that are significantly higher than the mean.