Solved

A Cellular Phone Company Wants to Locate Two New Communications $\quad$

question 50

Essay

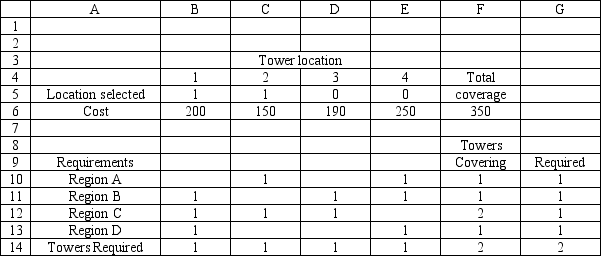
A cellular phone company wants to locate two new communications towers to cover 4 regions. The company wants to minimize the cost of installing the two towers. The regions that can be covered by each tower site are indicated by a 1 in the following table:
$\quad$ $\quad$ $\quad$ $\quad$ $\quad$ $\quad$ $\quad$ $\quad$ $\quad$ $\quad$ $\underline{\text { Tower Sites }}$
$\begin{array}{ccccc}\text { Region } & 1 & 2 & 3 & 4 \\\hline \text { A } & & 1 & & 1 \\\text { B } & 1 & & 1 & 1 \\\text { C } & 1 & 1 & 1 & \\\text { D } & 1 & & & 1 \\\hline \text { COST }(\$ 000 \mathrm{~s}) & 200 & 150 & 190 & 250\end{array}$

$\text { MIN: } \quad 200 \mathrm{X}_{1}+150 \mathrm{X}_{2}+190 \mathrm{X}_{3}+250 \mathrm{X}_{4}$

$\text { Subject to:}$
$\begin{array}{l}\mathrm{X}_{2}+\mathrm{X}_{4} \geq 1 \\\mathrm{X}_{1}+\mathrm{X}_{3}+\mathrm{X}_{4} \geq 1 \\\mathrm{X}_{1}+\mathrm{X}_{2}+\mathrm{X}_{3} \geq 1 \\\mathrm{X}_{1}+\mathrm{X}_{4} \geq 1 \\\mathrm{X}_{1}+\mathrm{X}_{2}+\mathrm{X}_{3}+\mathrm{X}_{4}=2 \\\mathrm{X}_{\mathrm{i}}=0,1\end{array}$ Based on this ILP formulation of the problem what formulas should go in cells F6:F14 of the following Excel spreadsheet?
$A cellular phone company wants to locate two new communications towers to cover 4 regions. The company wants to minimize the cost of installing the two towers. The regions that can be covered by each tower site are indicated by a 1 in the following table: \quad \quad \quad \quad \quad \quad \quad \quad \quad \quad \underline{\text { Tower Sites }} \begin{array}{ccccc} \text { Region } & 1 & 2 & 3 & 4 \\ \hline \text { A } & & 1 & & 1 \\ \text { B } & 1 & & 1 & 1 \\ \text { C } & 1 & 1 & 1 & \\ \text { D } & 1 & & & 1 \\ \hline \text { COST }(\$ 000 \mathrm{~s}) & 200 & 150 & 190 & 250 \end{array} \text { MIN: } \quad 200 \mathrm{X}_{1}+150 \mathrm{X}_{2}+190 \mathrm{X}_{3}+250 \mathrm{X}_{4} \text { Subject to:} \begin{array}{l} \mathrm{X}_{2}+\mathrm{X}_{4} \geq 1 \\ \mathrm{X}_{1}+\mathrm{X}_{3}+\mathrm{X}_{4} \geq 1 \\ \mathrm{X}_{1}+\mathrm{X}_{2}+\mathrm{X}_{3} \geq 1 \\ \mathrm{X}_{1}+\mathrm{X}_{4} \geq 1 \\ \mathrm{X}_{1}+\mathrm{X}_{2}+\mathrm{X}_{3}+\mathrm{X}_{4}=2 \\ \mathrm{X}_{\mathrm{i}}=0,1 \end{array} Based on this ILP formulation of the problem what formulas should go in cells F6:F14 of the following Excel spreadsheet?$

Comprehend principles related to leasing, renting, and homeownership, including understanding lease agreements.

Grasp the financial parameters involved in real estate investment, including equity, mortgage mechanics, and interest calculations.

Understand the importance and function of insurance and tax payments in homeownership.

Apply mathematical skills to real-world scenarios about property management, including area calculations and cost estimations.

Definitions:

Fair Coin

A theoretical coin which has an equal chance of landing on heads or tails.

Observed Frequencies

The actual number of occurrences or instances of a variable category observed in a statistical sample.

Chi-squared Goodness-of-fit Test

A statistical test used to determine if observed data fits a theoretical distribution.

Rule of Five

A statistical rule of thumb asserting that if a sample size is large enough (n>30), the sample means will be normally distributed.

A Cellular Phone Company Wants to Locate Two New Communications \quad

Definitions:

A Cellular Phone Company Wants to Locate Two New Communications $\quad$