Exhibit 7.4 The Following Questions Are Based on the Problem Below

question 56

Essay

Exhibit 7.4
The following questions are based on the problem below.
Robert Gardner runs a small, local-only delivery service. His fleet consists of three smaller panel trucks. He recently accepted a contract to deliver 12 shipping boxes of goods for delivery to 12 different customers. The box weights are: 210, 160, 320, 90, 110, 70, 410, 260, 170, 240, 80 and 180 for boxes 1 through 12, respectively. Since each truck differs each truck has different load capacities as given below: 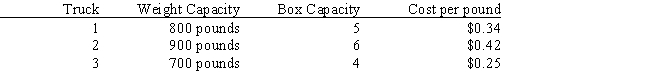Robert would like each truck equally loaded, both in terms of number of boxes and in terms of total weight, while minimizing his shipping costs. Assume a cost of $50 per item for trucks carrying extra boxes and $0.10 per pound cost for trucks carrying less weight.
The following integer goal programming formulation applies to his problem.
Y₁ = weight loaded in truck 1; Y₂ = weight loaded in truck 2; Y₃ = weight loaded in truck 3;
X_i,j = 0 if truck i not loaded with box j; 1 if truck i loaded with box j. Given the following spreadsheet solution of this integer goal programming formulation, answer the following questions.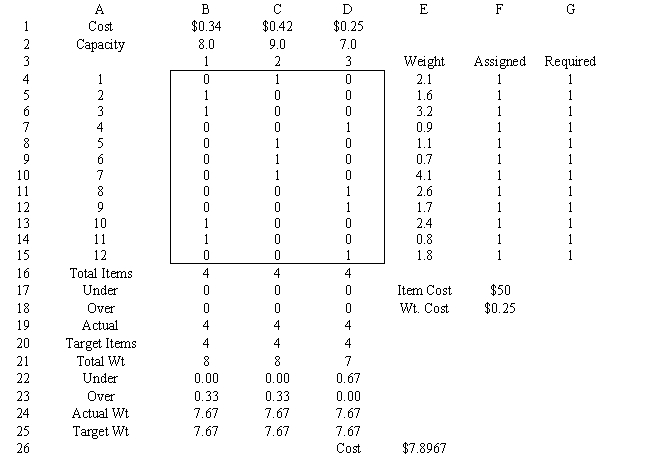
-Refer to Exhibit 7.4. Based on the integer goal programming formulation, the associated solution, and spreadsheet model, what formulas should go in cells B19:E19 and B24:E24 of the spreadsheet?

---

Definitions:

Economic Values

The worth of goods, services, or resources based on their utility and the benefits they provide to individuals or society.

Unethical Behaviour

Actions or practices that violate moral or professional guidelines and standards.

Stakeholders

Individuals or groups with an interest or concern in the success and operations of an organization or project.

Organizational Decisions

Choices made by individuals or groups within an organization that influence its activities and direction.