TABLE 13- 11
A company that has the distribution rights to home video sales of previously released movies would like to use the box office gross (in millions of dollars) to estimate the number of units (in thousands of units) that it can expect to sell. Following is the output from a simple linear regression along with the residual plot and normal probability plot obtained from a data set of 30 different movie titles:
ANOVA
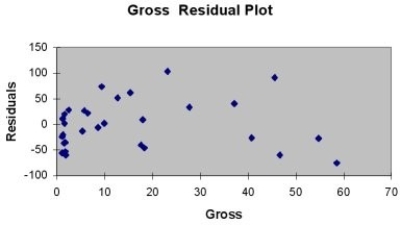
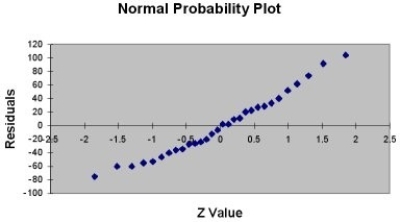
-Referring to Table 13-11, what is the critical value for testing whether there is a linear relationship between box office gross and home video unit sales at a 5% level of significance?
Definitions:
Dyadic Relationships
Two-person interactions or relationships, often analyzed in the context of leadership or management.
LMX
Leader-Member Exchange, a theory focusing on the two-way (dyadic) relationship between leaders and followers.
In-group Followers
Followers who are considered part of a leader's inner circle and typically enjoy closer relationships and more privileges than out-group members.
Leader-member Exchange Theory
A theory that emphasizes the quality of relationships between leaders and followers, proposing that better relationships lead to higher organizational outcomes.
Q13: Referring to Table 14-16, there is sufficient
Q20: The method of least squares may be
Q43: Referring to Table 13-10, construct a 95%
Q49: Referring to Table 12-12, if there is
Q64: When an additional explanatory variable is introduced
Q75: Referring to Table 11-11, the amount of
Q80: Referring to Table 15-9, what are, respectively,
Q89: Referring to Table 16-12, based on the
Q92: Referring to Table 13-12, the degrees of
Q128: Referring to Table 13-4, the managers of