TABLE 13- 11 a Company That Has the Distribution RightsANOVA-Referring to Table 13-11, Predict the Video Unit Sales for to Home

The Answer of TABLE 13- 11 a Company That Has the Distribution RightsANOVA-Referring to Table 13-11, Predict the Video Unit Sales for to Home

TABLE 13- 11 a Company That Has the Distribution RightsANOVA-Referring to Table 13-11, Predict the Video Unit Sales for to Home

The Answer of TABLE 13- 11 a Company That Has the Distribution RightsANOVA-Referring to Table 13-11, Predict the Video Unit Sales for to Home

Solved

TABLE 13- 11
a Company That Has the Distribution Rights

question 191

Short Answer

TABLE 13- 11
A company that has the distribution rights to home video sales of previously released movies would like to use the box office gross (in millions of dollars) to estimate the number of units (in thousands of units) that it can expect to sell. Following is the output from a simple linear regression along with the residual plot and normal probability plot obtained from a data set of 30 different movie titles:
$\begin{array}{lc}\hline \text { Regression Statistics } & \\\hline \text { Multiple R } & 0.8531 \\\text { R Square } & 0.7278 \\\text { Adjusted R Square } & 0.7180 \\\text { Standard Error } & 47.8668 \\\text { Observations } & 30\end{array}$

ANOVA
$\begin{array}{lrrrrr}\hline &\text { d f}& \text { SS } & \text { MS } & \text {F }& \text {Significance F} \\\hline \text {Regression }& 1 & 171499.78 & 171499.78 & 74.8505 & 2.1259E-09 \\\text {Residual} & 28 & 64154.42 & 2291.23 & & \\\text {Total} & 29 & 235654.20 & & & \\\hline\end{array}$

$\begin{array}{lrrrrrr}\hline & \text {Coefficients }& \text { Standard Error}& \text { t Stat }& \text { p -value }& \text {Lower 95\% }& \text {Upper 95\% }\\\hline \text { Intercept }& 76.5351 & 11.8318 & 6.4686 & 5.24 \mathrm{E}-07& 52.2987 & 100.7716 \\ \text {Gross} & 4.3331 & 0.5008 & 8.6516 & 2.13 \mathrm{E}-09 & 3.3072 & 5.3590 \\\hline\end{array}$

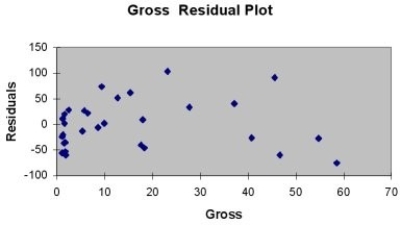
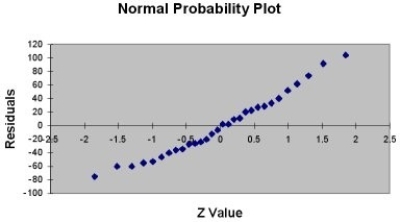
$TABLE 13- 11 A company that has the distribution rights to home video sales of previously released movies would like to use the box office gross (in millions of dollars) to estimate the number of units (in thousands of units) that it can expect to sell. Following is the output from a simple linear regression along with the residual plot and normal probability plot obtained from a data set of 30 different movie titles: \begin{array}{lc} \hline \text { Regression Statistics } & \\ \hline \text { Multiple R } & 0.8531 \\ \text { R Square } & 0.7278 \\ \text { Adjusted R Square } & 0.7180 \\ \text { Standard Error } & 47.8668 \\ \text { Observations } & 30 \end{array} ANOVA \begin{array}{lrrrrr} \hline &\text { d f}& \text { SS } & \text { MS } & \text {F }& \text {Significance F} \\ \hline \text {Regression }& 1 & 171499.78 & 171499.78 & 74.8505 & 2.1259E-09 \\ \text {Residual} & 28 & 64154.42 & 2291.23 & & \\ \text {Total} & 29 & 235654.20 & & & \\ \hline\end{array} \begin{array}{lrrrrrr} \hline & \text {Coefficients }& \text { Standard Error}& \text { t Stat }& \text { p -value }& \text {Lower 95\% }& \text {Upper 95\% }\\ \hline \text { Intercept }& 76.5351 & 11.8318 & 6.4686 & 5.24 \mathrm{E}-07& 52.2987 & 100.7716 \\ \text {Gross} & 4.3331 & 0.5008 & 8.6516 & 2.13 \mathrm{E}-09 & 3.3072 & 5.3590 \\ \hline \end{array} -Referring to Table 13-11, predict the video unit sales for a movie that had a box office gross of $30 millions.$ $TABLE 13- 11 A company that has the distribution rights to home video sales of previously released movies would like to use the box office gross (in millions of dollars) to estimate the number of units (in thousands of units) that it can expect to sell. Following is the output from a simple linear regression along with the residual plot and normal probability plot obtained from a data set of 30 different movie titles: \begin{array}{lc} \hline \text { Regression Statistics } & \\ \hline \text { Multiple R } & 0.8531 \\ \text { R Square } & 0.7278 \\ \text { Adjusted R Square } & 0.7180 \\ \text { Standard Error } & 47.8668 \\ \text { Observations } & 30 \end{array} ANOVA \begin{array}{lrrrrr} \hline &\text { d f}& \text { SS } & \text { MS } & \text {F }& \text {Significance F} \\ \hline \text {Regression }& 1 & 171499.78 & 171499.78 & 74.8505 & 2.1259E-09 \\ \text {Residual} & 28 & 64154.42 & 2291.23 & & \\ \text {Total} & 29 & 235654.20 & & & \\ \hline\end{array} \begin{array}{lrrrrrr} \hline & \text {Coefficients }& \text { Standard Error}& \text { t Stat }& \text { p -value }& \text {Lower 95\% }& \text {Upper 95\% }\\ \hline \text { Intercept }& 76.5351 & 11.8318 & 6.4686 & 5.24 \mathrm{E}-07& 52.2987 & 100.7716 \\ \text {Gross} & 4.3331 & 0.5008 & 8.6516 & 2.13 \mathrm{E}-09 & 3.3072 & 5.3590 \\ \hline \end{array} -Referring to Table 13-11, predict the video unit sales for a movie that had a box office gross of $30 millions.$
-Referring to Table 13-11, predict the video unit sales for a movie that had a box office gross of $30 millions.

Definitions:

Non-Unionized Business

A workplace where the workforce is not represented by a labor union, affecting collective bargaining and worker rights.

Employment Contract

A formal agreement between an employer and employee that outlines the terms and conditions of employment.

Common Law

refers to law derived from judicial decisions of courts and similar tribunals, as opposed to statutes or regulations.

Employment Standards Act

A body of law that establishes minimum employment conditions such as wages, hours of work, and leave entitlements.