TABLE 13- 11 a Company That Has the Distribution RightsANOVA-Referring to Table 13-11, What Is the Value of the to Home

The Answer of TABLE 13- 11 a Company That Has the Distribution RightsANOVA-Referring to Table 13-11, What Is the Value of the to Home

TABLE 13- 11 a Company That Has the Distribution RightsANOVA-Referring to Table 13-11, What Is the Value of the to Home

The Answer of TABLE 13- 11 a Company That Has the Distribution RightsANOVA-Referring to Table 13-11, What Is the Value of the to Home

Solved

TABLE 13- 11
a Company That Has the Distribution Rights

question 186

Short Answer

TABLE 13- 11
A company that has the distribution rights to home video sales of previously released movies would like to use the box office gross (in millions of dollars) to estimate the number of units (in thousands of units) that it can expect to sell. Following is the output from a simple linear regression along with the residual plot and normal probability plot obtained from a data set of 30 different movie titles:
$\begin{array}{l}\text { Regression Statistics }\\\begin{array} { l c } \hline \text { Multiple R } & 0.8531 \\\text { RSquare } & 0.7278 \\\text { Adjusted R Square } & 0.7180 \\\text { Standard Error } & 47.8668 \\\text { Observations } & 30\end{array}\end{array}$
ANOVA
$\begin{array}{lrrrrr}\hline &\text { d f}& \text { SS } & \text { MS } & \text {F }& \text {Significance F} \\\hline \text {Regression }& 1 & 171499.78 & 171499.78 & 74.8505 & 2.1259E-09 \\\text {Residual} & 28 & 64154.42 & 2291.23 & & \\\text {Total} & 29 & 235654.20 & & & \\\hline\end{array}$

$\begin{array}{lrrrrrr}\hline & \text {Coefficients }& \text { Standard Error}& \text { t Stat }& \text { p -value }& \text {Lower 95\% }& \text {Upper 95\% }\\\hline \text { Intercept }& 76.5351 & 11.8318 & 6.4686 & 5.24 \mathrm{E}-07& 52.2987 & 100.7716 \\ \text {Gross} & 4.3331 & 0.5008 & 8.6516 & 2.13 \mathrm{E}-09 & 3.3072 & 5.3590 \\\hline\end{array}$

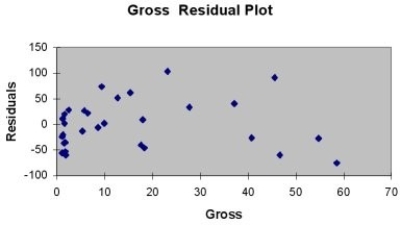
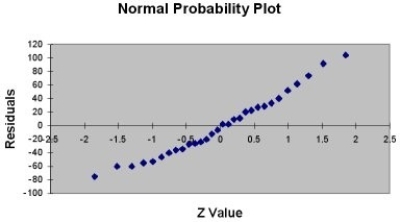
$TABLE 13- 11 A company that has the distribution rights to home video sales of previously released movies would like to use the box office gross (in millions of dollars) to estimate the number of units (in thousands of units) that it can expect to sell. Following is the output from a simple linear regression along with the residual plot and normal probability plot obtained from a data set of 30 different movie titles: \begin{array}{l} \text { Regression Statistics }\\ \begin{array} { l c } \hline \text { Multiple R } & 0.8531 \\ \text { RSquare } & 0.7278 \\ \text { Adjusted R Square } & 0.7180 \\ \text { Standard Error } & 47.8668 \\ \text { Observations } & 30 \end{array} \end{array} ANOVA \begin{array}{lrrrrr} \hline &\text { d f}& \text { SS } & \text { MS } & \text {F }& \text {Significance F} \\ \hline \text {Regression }& 1 & 171499.78 & 171499.78 & 74.8505 & 2.1259E-09 \\ \text {Residual} & 28 & 64154.42 & 2291.23 & & \\ \text {Total} & 29 & 235654.20 & & & \\ \hline\end{array} \begin{array}{lrrrrrr} \hline & \text {Coefficients }& \text { Standard Error}& \text { t Stat }& \text { p -value }& \text {Lower 95\% }& \text {Upper 95\% }\\ \hline \text { Intercept }& 76.5351 & 11.8318 & 6.4686 & 5.24 \mathrm{E}-07& 52.2987 & 100.7716 \\ \text {Gross} & 4.3331 & 0.5008 & 8.6516 & 2.13 \mathrm{E}-09 & 3.3072 & 5.3590 \\ \hline \end{array} -Referring to Table 13-11, what is the value of the test statistic for testing whether there is a linear relationship between box office gross and home video unit sales?$ $TABLE 13- 11 A company that has the distribution rights to home video sales of previously released movies would like to use the box office gross (in millions of dollars) to estimate the number of units (in thousands of units) that it can expect to sell. Following is the output from a simple linear regression along with the residual plot and normal probability plot obtained from a data set of 30 different movie titles: \begin{array}{l} \text { Regression Statistics }\\ \begin{array} { l c } \hline \text { Multiple R } & 0.8531 \\ \text { RSquare } & 0.7278 \\ \text { Adjusted R Square } & 0.7180 \\ \text { Standard Error } & 47.8668 \\ \text { Observations } & 30 \end{array} \end{array} ANOVA \begin{array}{lrrrrr} \hline &\text { d f}& \text { SS } & \text { MS } & \text {F }& \text {Significance F} \\ \hline \text {Regression }& 1 & 171499.78 & 171499.78 & 74.8505 & 2.1259E-09 \\ \text {Residual} & 28 & 64154.42 & 2291.23 & & \\ \text {Total} & 29 & 235654.20 & & & \\ \hline\end{array} \begin{array}{lrrrrrr} \hline & \text {Coefficients }& \text { Standard Error}& \text { t Stat }& \text { p -value }& \text {Lower 95\% }& \text {Upper 95\% }\\ \hline \text { Intercept }& 76.5351 & 11.8318 & 6.4686 & 5.24 \mathrm{E}-07& 52.2987 & 100.7716 \\ \text {Gross} & 4.3331 & 0.5008 & 8.6516 & 2.13 \mathrm{E}-09 & 3.3072 & 5.3590 \\ \hline \end{array} -Referring to Table 13-11, what is the value of the test statistic for testing whether there is a linear relationship between box office gross and home video unit sales?$

-Referring to Table 13-11, what is the value of the test statistic for testing whether there is a linear relationship between box office gross and home video unit sales?

Understand the concepts related to change leadership and its role in organizational development.

Differentiate between change leadership and status quo management.

Recognize the types of organizational changes and their implications for performance.

Appreciate the role of a manager as a change agent in fostering diversity, inclusion, and organizational change.

Definitions:

Preterm

The term for babies born before the 37th week of pregnancy.

Cell Division

The process by which a parent cell divides into two or more daughter cells, crucial for growth, reproduction, and repair in living organisms.

Embryo

The early stage of development in multicellular organisms, in humans, it refers to the period from fertilization until the end of the eighth week of gestation, when the being is then referred to as a fetus.

Preterm Birth Rate

The proportion of births that occur before a pregnancy reaches full term, often defined as before 37 weeks of gestation.