TABLE 13- 11
A company that has the distribution rights to home video sales of previously released movies would like to use the box office gross (in millions of dollars) to estimate the number of units (in thousands of units) that it can expect to sell. Following is the output from a simple linear regression along with the residual plot and normal probability plot obtained from a data set of 30 different movie titles:
ANOVA
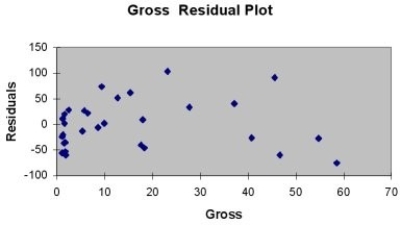
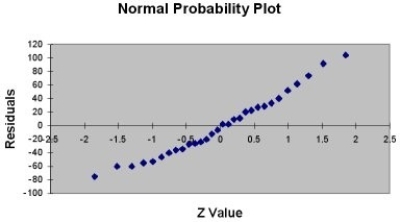
-Referring to Table 13-11, there is sufficient evidence that box office gross and home video unit sales are linearly related at a 5% level of significance.
Definitions:
Norm Intensity
The degree to which a norm is accepted and enforced within a group or organization, affecting members' behaviors and attitudes.
Cohesiveness
The degree to which members of a group or team are united in pursuing common goals and how well they bond with each other.
Social Behaviour
Actions among individuals, shaped by social norms, cultures, and societal structures, that affect and are affected by others.
Q4: The sample correlation coefficient between X and
Q11: Referring to Table 14-3, what is the
Q18: Referring to Table 11-10, based on the
Q43: Referring to Table 14-1, if an employee
Q55: Referring to Table 14-3, one economy in
Q68: Referring to Table 11-4, at 1% level
Q73: Referring to Table 11-4, the F test
Q79: Referring to Table 13-10, the residual plot
Q99: When the F test is used for
Q244: Consider a regression in which b<sub>2</sub> =