You Have Collected Data from Major League Baseball (MLB)to Find $\widehat { \text { Winpct } } = 0.94 - 0.100 \times \text { teamera } , \quad R ^ { 2 } = 0.49 , S E R = 0.06 \text {. }$

question 1

Essay

You have collected data from Major League Baseball (MLB)to find the determinants of
winning.You have a general idea that both good pitching and strong hitting are needed to do
well.However, you do not know how much each of these contributes separately.To
investigate this problem, you collect data for all MLB during 1999 season.Your strategy is to
first regress the winning percentage on pitching quality ("Team ERA"), second to regress the
same variable on some measure of hitting ("OPS - On-base Plus Slugging percentage"), and
third to regress the winning percentage on both. Summary of the Distribution of Winning Percentage, On Base plus
Slugging percentage, and Team Earned Run Average for MLB in 1999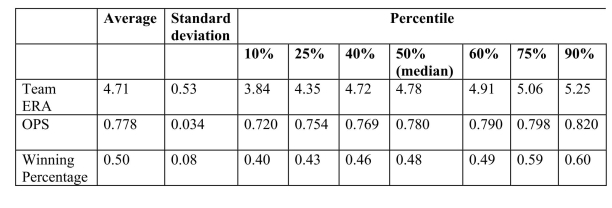

The results are as follows:
$\widehat { \text { Winpct } } = 0.94 - 0.100 \times \text { teamera } , \quad R ^ { 2 } = 0.49 , S E R = 0.06 \text {. }$

$\widehat { \text { Winpct } } = - 0.68 + 1.513 \times$ ops, $R ^ { 2 } = 0.45$ , SER $= 0.06$ .

$$\widehat { \text { Winpct } } = - 0.19 - 0.099 \times \text { teamera } + 1.490 \times \text { ops } , R ^ { 2 } = 0.92 , S E R = 0.02 \text {. }$$ (a)Interpret the multiple regression.What is the effect of a one point increase in team ERA?
Given that the Atlanta Braves had the most wins that year, wining 103 games out of 162,
do you find this effect important? Next analyze the importance and statistical significance
for the OPS coefficient.(The Minnesota Twins had the minimum OPS of 0.712, while
the Texas Rangers had the maximum with 0.840.)Since the intercept is negative, and
since winning percentages must lie between zero and one, should you rerun the
regression through the origin?

---

Definitions:

Machining

The process of removing material from a workpiece to shape or finish it using machine tools.

Manufacturing Overhead

All indirect costs associated with the production process, excluding direct material and direct labor costs.

Predetermined Overhead Rate

A rate established in advance to allocate overhead costs to products or services based on a specific activity base.

Machine-Hours

A measure of production time where one machine-hour equals the operation of one machine for one hour.