SCENARIO 16-13 Given Below Is the Monthly Time Series DataThe Results of the Linear Trend, Quadratic Trend, Exponential Trend

The Answer of SCENARIO 16-13 Given Below Is the Monthly Time Series DataThe Results of the Linear Trend, Quadratic Trend, Exponential Trend

SCENARIO 16-13 Given Below Is the Monthly Time Series DataThe Results of the Linear Trend, Quadratic Trend, Exponential Trend

The Answer of SCENARIO 16-13 Given Below Is the Monthly Time Series DataThe Results of the Linear Trend, Quadratic Trend, Exponential Trend

Solved

SCENARIO 16-13
Given Below Is the Monthly Time Series Data

question 21

Short Answer

SCENARIO 16-13
Given below is the monthly time series data for U.S. retail sales of building materials over a
specific year. $\begin{array} { | c | c | } \hline \text { Month } & \text { Retail Sales } \\\hline 1 & 6,594 \\\hline 2 & 6,610 \\\hline 3 & 8,174 \\\hline 4 & 9,513 \\\hline 5 & 10,595 \\\hline 6 & 10,415 \\\hline 7 & 9,949 \\\hline 8 & 9,810 \\\hline 9 & 9,637 \\\hline 10 & 9,732 \\\hline 11 & 9,214 \\\hline 12 & 9,201 \\\hline\end{array}$ The results of the linear trend, quadratic trend, exponential trend, first-order autoregressive,
second-order autoregressive and third-order autoregressive model are presented below in which
the coded month for the 1st month is 0: $\text { Linear trend model: }$
$\begin{array}{lrrrr} & \text { Coefficients } & \text { Standard Error } & \text { t Stat } & \text { P-value } \\\hline \text { Intercept } & 7950.7564 & 617.6342 & 12.8729 & 0.0000 \\\text { Coded Month } & 212.6503 & 95.1145 & 2.2357 & 0.0494\end{array}$

$\text { Quadratic trend model: }$
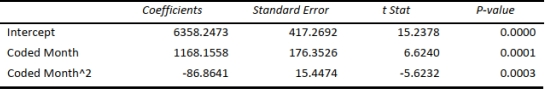
$SCENARIO 16-13 Given below is the monthly time series data for U.S. retail sales of building materials over a specific year. \begin{array} { | c | c | } \hline \text { Month } & \text { Retail Sales } \\ \hline 1 & 6,594 \\ \hline 2 & 6,610 \\ \hline 3 & 8,174 \\ \hline 4 & 9,513 \\ \hline 5 & 10,595 \\ \hline 6 & 10,415 \\ \hline 7 & 9,949 \\ \hline 8 & 9,810 \\ \hline 9 & 9,637 \\ \hline 10 & 9,732 \\ \hline 11 & 9,214 \\ \hline 12 & 9,201 \\ \hline \end{array} The results of the linear trend, quadratic trend, exponential trend, first-order autoregressive, second-order autoregressive and third-order autoregressive model are presented below in which the coded month for the 1st month is 0: \text { Linear trend model: } \begin{array}{lrrrr} & \text { Coefficients } & \text { Standard Error } & \text { t Stat } & \text { P-value } \\ \hline \text { Intercept } & 7950.7564 & 617.6342 & 12.8729 & 0.0000 \\ \text { Coded Month } & 212.6503 & 95.1145 & 2.2357 & 0.0494 \end{array} \text { Quadratic trend model: } \text { Exponential trend model: } \begin{array}{lrrrr} \hline & \text { Coefficients } & \text { Standard Error } & \text { t Stat } & \text { P-value } \\ \hline \text { Intercept } & 3.8912 & 0.0315 & 123.3674 & 0.0000 \\ \text { Coded Month } & 0.0116 & 0.0049 & 2.3957 & 0.0376 \end{array} \text { First-order autoregressive: } \begin{array}{lrrrr} & \text { Coefficients } & \text { Standard Error } & t \text { Stat } & {\text { P-value }} \\ \hline \text { Intercept } & 3132.0951 & 1287.2899 & 2.4331 & 0.0378 \\ \text { YLag1 } & 0.6823 & 0.1398 & 4.8812 & 0.0009 \\ \hline \end{array} -Referring to Scenario 16-13, what is your forecast for the 13 ^ { \text {th } } month using the first-order autoregressive model?$

$\text { Exponential trend model: }$
$\begin{array}{lrrrr}\hline & \text { Coefficients } & \text { Standard Error } & \text { t Stat } & \text { P-value } \\\hline \text { Intercept } & 3.8912 & 0.0315 & 123.3674 & 0.0000 \\\text { Coded Month } & 0.0116 & 0.0049 & 2.3957 & 0.0376\end{array}$

$\text { First-order autoregressive: }$
$\begin{array}{lrrrr} & \text { Coefficients } & \text { Standard Error } & t \text { Stat } & {\text { P-value }} \\\hline \text { Intercept } & 3132.0951 & 1287.2899 & 2.4331 & 0.0378 \\\text { YLag1 } & 0.6823 & 0.1398 & 4.8812 & 0.0009 \\\hline\end{array}$

-Referring to Scenario 16-13, what is your forecast for the $13 ^ { \text {th } }$ month using the first-order
autoregressive model?

Definitions:

Positive Effect

A beneficial impact or outcome resulting from a particular action or set of conditions.

Connotative Meaning

The meaning of a word or phrase that is based on past personal experiences or associations.

Manipulate Others

The act of influencing or controlling someone's behavior or emotions for one's own purposes, often without their knowledge.

Language

A system of communication used by a particular community or country, consisting of written, spoken, or signed words and the rules for combining them to convey meaning.