SCENARIO 16-13 Given Below Is the Monthly Time Series DataThe Results of the Linear Trend, Quadratic Trend, Exponential Trend

The Answer of SCENARIO 16-13 Given Below Is the Monthly Time Series DataThe Results of the Linear Trend, Quadratic Trend, Exponential Trend

SCENARIO 16-13 Given Below Is the Monthly Time Series DataThe Results of the Linear Trend, Quadratic Trend, Exponential Trend

The Answer of SCENARIO 16-13 Given Below Is the Monthly Time Series DataThe Results of the Linear Trend, Quadratic Trend, Exponential Trend

Solved

SCENARIO 16-13
Given Below Is the Monthly Time Series Data

question 73

Short Answer

SCENARIO 16-13
Given below is the monthly time series data for U.S. retail sales of building materials over a
specific year. $\begin{array} { | c | c | } \hline \text { Month } & \text { Retail Sales } \\\hline 1 & 6,594 \\\hline 2 & 6,610 \\\hline 3 & 8,174 \\\hline 4 & 9,513 \\\hline 5 & 10,595 \\\hline 6 & 10,415 \\\hline 7 & 9,949 \\\hline 8 & 9,810 \\\hline 9 & 9,637 \\\hline 10 & 9,732 \\\hline 11 & 9,214 \\\hline 12 & 9,201 \\\hline\end{array}$ The results of the linear trend, quadratic trend, exponential trend, first-order autoregressive,
second-order autoregressive and third-order autoregressive model are presented below in which
the coded month for the 1st month is 0: $\text { Linear trend model: }$
$\begin{array}{lrrrr} & \text { Coefficients } & \text { Standard Error } & \text { t Stat } & \text { P-value } \\\hline \text { Intercept } & 7950.7564 & 617.6342 & 12.8729 & 0.0000 \\\text { Coded Month } & 212.6503 & 95.1145 & 2.2357 & 0.0494\end{array}$

$\text { Quadratic trend model: }$
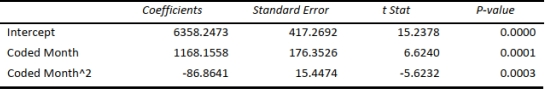
$SCENARIO 16-13 Given below is the monthly time series data for U.S. retail sales of building materials over a specific year. \begin{array} { | c | c | } \hline \text { Month } & \text { Retail Sales } \\ \hline 1 & 6,594 \\ \hline 2 & 6,610 \\ \hline 3 & 8,174 \\ \hline 4 & 9,513 \\ \hline 5 & 10,595 \\ \hline 6 & 10,415 \\ \hline 7 & 9,949 \\ \hline 8 & 9,810 \\ \hline 9 & 9,637 \\ \hline 10 & 9,732 \\ \hline 11 & 9,214 \\ \hline 12 & 9,201 \\ \hline \end{array} The results of the linear trend, quadratic trend, exponential trend, first-order autoregressive, second-order autoregressive and third-order autoregressive model are presented below in which the coded month for the 1st month is 0: \text { Linear trend model: } \begin{array}{lrrrr} & \text { Coefficients } & \text { Standard Error } & \text { t Stat } & \text { P-value } \\ \hline \text { Intercept } & 7950.7564 & 617.6342 & 12.8729 & 0.0000 \\ \text { Coded Month } & 212.6503 & 95.1145 & 2.2357 & 0.0494 \end{array} \text { Quadratic trend model: } \text { Exponential trend model: } \begin{array}{lrrrr} \hline & \text { Coefficients } & \text { Standard Error } & \text { t Stat } & \text { P-value } \\ \hline \text { Intercept } & 3.8912 & 0.0315 & 123.3674 & 0.0000 \\ \text { Coded Month } & 0.0116 & 0.0049 & 2.3957 & 0.0376 \end{array} \text { First-order autoregressive: } \begin{array}{lrrrr} & \text { Coefficients } & \text { Standard Error } & t \text { Stat } & {\text { P-value }} \\ \hline \text { Intercept } & 3132.0951 & 1287.2899 & 2.4331 & 0.0378 \\ \text { YLag1 } & 0.6823 & 0.1398 & 4.8812 & 0.0009 \\ \hline \end{array} -Referring to Scenario 16-13, what is your forecast for the 13 ^ { \text {th } } month using the linear-trend model?$

$\text { Exponential trend model: }$
$\begin{array}{lrrrr}\hline & \text { Coefficients } & \text { Standard Error } & \text { t Stat } & \text { P-value } \\\hline \text { Intercept } & 3.8912 & 0.0315 & 123.3674 & 0.0000 \\\text { Coded Month } & 0.0116 & 0.0049 & 2.3957 & 0.0376\end{array}$

$\text { First-order autoregressive: }$
$\begin{array}{lrrrr} & \text { Coefficients } & \text { Standard Error } & t \text { Stat } & {\text { P-value }} \\\hline \text { Intercept } & 3132.0951 & 1287.2899 & 2.4331 & 0.0378 \\\text { YLag1 } & 0.6823 & 0.1398 & 4.8812 & 0.0009 \\\hline\end{array}$

-Referring to Scenario 16-13, what is your forecast for the $13 ^ { \text {th } }$ month using the linear-trend
model?

Definitions:

Antibodies

Proteins in the blood that identify and neutralize foreign substances like bacteria and viruses.

Kiecolt-Glaser

Refers to psychologist Janice Kiecolt-Glaser, known for her research on stress and its effects on the human immune system.

Alzheimer's Caregivers

Individuals who provide care and support to those with Alzheimer's disease, often involving daily assistance, emotional support, and managing medications or therapy.

Immune System

The complex network of cells, tissues, and organs that protects the body against infections and diseases.