SCENARIO 14-15
the Superintendent of a School District Wanted to Predict

question 196

True/False

SCENARIO 14-15
The superintendent of a school district wanted to predict the percentage of students passing a sixth-
grade proficiency test. She obtained the data on percentage of students passing the proficiency test
(% Passing), mean teacher salary in thousands of dollars (Salaries), and instructional spending per
pupil in thousands of dollars (Spending) of 47 schools in the state. Following is the multiple regression output with $Y = \%$ Passing as the dependent variable, $X _ { 1 } =$
Salaries and $X _ { 2 } =$ Spending:

$\begin{array}{lr}\hline {\text { Regression Statistics }} \\\hline \text { Multiple R } & 0.4276 \\\text { R Square } & 0.1828 \\\text { Adjusted R Square } & 0.1457 \\\text { Standard Error } & 5.7351 \\\text { Observations } & 47 \\\hline\end{array}$

ANOVA


$\begin{array}{lrrrrrr}\hline & \text { Coefficients } & \text { Standard Error } & \text { t Stat } & \rho \text {-value } & \text { Lower 95\% } & \text { Upper 95\% } \\\hline \text { Intercept } & -72.9916 & 45.9106 & -1.5899 & 0.1190 & -165.5184 & 19.5352 \\\text { Salary } & 2.7939 & 0.8974 & 3.1133 & 0.0032 & 0.9853 & 4.6025 \\\text { Spending } & 0.3742 & 0.9782 & 0.3825 & 0.7039 & -1.5972 & 2.3455 \\\hline\end{array}$

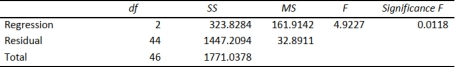
-Referring to Scenario 14-15, the null hypothesis $H _ { 0 } : \beta _ { 1 } = \beta _ { 2 } = 0$ implies that percentage of
students passing the proficiency test is not related to one of the explanatory variables.

---

Definitions:

Trimalleolar Fracture

A type of ankle fracture that involves all three malleoli of the ankle bones.

Displaced

Refers to something forcefully moved or put out of its usual or proper place, often used in medical contexts, like bone fractures.

Lower Leg

The part of the leg between the knee and the ankle, comprising the tibia and fibula bones.

Depressed Fracture

A type of bone fracture where part of the skull is sunken in due to trauma.